Preparation and Quasi-Isentropic Loading Characteristics of Ti-Pt Periodically Modulated Gradient Material
-
摘要: 基于波阻抗梯度材料的准等熵加载技术是掌握材料动态响应特性的重要技术手段,对于提升材料服役性能至关重要。采用电子束蒸发镀膜技术成功制备出Ti-Pt周期调制梯度材料,通过对周期层内双组分(Ti和Pt单层)厚度的调控,实现了波阻抗的宏观梯度变化。梯度材料实测总厚度与理论设计总厚度的误差仅为1.67%,并且实测平均硬度及弹性模量分别为2.8和99.8 GPa。材料内部层界面清晰,物相分析未发现金属合金相。利用一级轻气炮驱动Ti-Pt周期调制梯度材料加载5 μm厚Al靶,在Al靶内产生冲击-准等熵加载波形。数值模拟结果与实验曲线在上升趋势上吻合良好,5 μm厚Al靶处的粒子速度、应力和应变率曲线的准等熵段存在较大起伏,应变率曲线在正负值间持续振荡,并且振幅较大。应力云图显示周期调制梯度材料在加载过程中会形成多个波系的追赶、叠加、整合。数值模拟结果显示,当靶材厚度为60 μm时,波系完成整合,转变为连续的压缩波。结合数值模拟结果开展了60 μm厚Al靶的轻气炮加载实验,粒子速度曲线和应力曲线的准等熵段转变为平滑的加载波形,应变率曲线准等熵段振幅显著减小,实现了良好的准等熵加载效果。研究结果表明,周期调制梯度材料与靶材厚度需进行匹配设计,研究结果可为新型周期调制梯度结构的应用提供指导。
-
关键词:
- Ti-Pt周期调制梯度材料 /
- 电子束蒸发镀膜 /
- 准等熵加载 /
- 波系分析
Abstract: The quasi-isentropic loading technique based on wave impedance gradient materials is a crucial method for understanding the dynamic response characteristics of materials, which is essential for enhancing material service performance. In this study, Ti-Pt periodically modulated gradient materials were successfully prepared using electron beam evaporation deposition technology. By adjusting the thickness of the two components (Ti and Pt single layers) within the periodic layers, a macroscopic gradient in wave impedance was achieved. The total thickness error between the measured gradient material and the theoretical design was only 1.67%, with an average hardness and elastic modulus of 2.8 and 99.8 GPa, respectively. The interfaces between the internal layers of the material were clear, and no metal alloy phases were detected in the phase analysis. The Ti-Pt periodically modulated gradient material was loaded onto a 5 μm-thickness Al target by a one-stage light gas gun, generating a shock-quasi-isentropic loading waveform within the Al target. Numerical simulation results showed good agreement in the rising trend with the experimental curve. For the 5 μm-thickness Al target, the particle velocity, stress, and strain rate curves exhibit significant fluctuations in the quasi-isentropic stage, with the strain rate curve oscillating continuously between positive and negative values with large amplitudes. Stress contour maps indicate that the loading process of the periodically modulated gradient material involved the chasing, superposition, and integration of multiple wave systems. Simulation shows that when the target thickness is 60 μm, the wave systems complete integration and change into continuous compression wave loading. Based on the simulation result, light gas gun loading experiment for the Al target with a thickness of 60 μm were conducted. The particle velocity and stress curves in the quasi-isentropic stage change into smooth loading waveforms, and the strain rate curve remains overall positive, achieving a good quasi-isentropic loading. This indicates that the periodically modulated gradient material and target thickness need to be designed to match each other. The results of this study provide a guidance for the application of novel periodically modulated gradient structures. -
国防装备的进步和工程技术的发展离不开材料服役性能的提升。在安全防护、穿甲侵彻、空间碎片撞击、爆炸焊接和高速切削摩擦等真实服役环境下,核心材料往往会经历极高应力和高应变率的动态加载作用[1–2]。材料的动力学响应行为,如相变行为[3]和强度特性[4]等,与加载过程中的应变率有很强的关联性,即材料具有“率效应”[5]。研究和掌握材料在动态加载过程中对外部应力载荷的响应特性,如弹-塑性变形、屈服、断裂、层裂失效相变等动力学行为,对于材料的设计和性能提升至关重要。
目前,多种加载技术可实现不同的应力和应变率范围,对应材料的不同服役环境。静高压加载技术能够在一定范围内调控加载压力和温度,但应变率较低(10−4~1 s−1);霍普金森杆(Hopkinson bar,HB)加载方法获得的加载应力(小于100 MPa)、应变率(1~103 s−1)也相对较低[6];冲击加载技术虽然能产生极端高压(1~100 GPa)、极高的应变率(107~109 s−1),但应力、应变率与温度存在强耦合关系,无法单独调控应力或应变率[7]。基于准等熵加载原理的可控加载技术,可单独调控应力(1~100 GPa)或应变率(104~107 s−1),是各国大力发展的动态加载技术[8–10]。
波阻抗梯度材料是多级炮准等熵加载技术的核心。自1984年至今,梯度材料研究已经有30余年历史,国内外多家实验室对叠层组分型梯度材料的设计、制备及其应用研究进行了诸多探索。早期的波阻抗梯度材料一般由数种不同厚度、不同密度的金属/聚合物薄片叠合粘结而成,如Mg/Al/Ti/Cu、Lexan/Al/Ti/Cu/Ta等多层组合式梯度飞片,与应变率大于107 s−1条件下的冲击加载相比,这些飞片的准等熵加载段应变率降低了3~4个数量级,约为103~104 s−1,但由于其相邻过渡层间的波阻抗跃变幅度大、结合强度低,击靶产生的加载波形呈阶梯状上升,应变率波形出现大幅振荡。2006年,Nguyen等[8]采用粉末冶金方法制备Al(Mg)-W飞片加载Cu靶,实现的应变率范围为104~106 s−1,应力范围达吉帕量级。2007年美国劳伦斯利弗莫尔国家实验室(Lawrence Livermore National Laboratory,LLNL)的Martin等[11]混合PE/Mg粉末制备了20层密度准连续的梯度飞片,在气炮加载实验中获得了更加光滑连续的加载斜波,靶材应变率范围达104~106 s−1。2018年,Tan等[12]设计了Ti-Cu-W梯度飞片,采用多级炮加载方法撞击Bi和LY12Al,实现了30~50 GPa、105~106 s−1的可控加载应力与应变率。
从结构形式上看,这些多层粘结式梯度飞片、准连续梯度飞片,都属于叠层组分型,通过中间过渡层数、前后端面密度和相邻层间的密度差异来调控梯度材料的密度分布,击靶后可获得不同的初始压力、峰值压力以及应变率等加载参数,实现对靶样品的可控加载。
叠层组分型梯度材料的优势在于能够独立调控过渡层的层数、组分、厚度以及密度范围、密度分布形式。但是,层层堆叠、组合制备得到的叠层组分型梯度材料在各过渡层之间通过胶粘、烧结等方式结合,结合界面不可避免地存在裂纹、孔洞、杂相以及低平行度、低平整度等问题,从而影响加载波形的连续性和一致性。2007年LLNL的Bastea等[13]通过数值模拟研究首次提出准等熵加载过程中的一些因素(胶粘、样品厚度控制精度、材料波阻抗分布曲线以及原位诊断技术等)对被测材料状态方程(equation of state,EOS)的影响,其中某些因素的细小变化会导致EOS测量过程中产生误差,如胶粘层的厚度变化导致3%~10%的误差。
LLNL的Kelly等[14]探究了采用流延法制备的Al-Cu-W-Ta叠层梯度飞片的密度、波阻抗的理论设计值与实际值间差距的原因,发现流延法使用的金属粉末存在氧化,烧结不致密,烧结过程中不可避免地生成金属间化合物等问题,均会影响材料的实际波阻抗。因此,如何提升梯度材料过渡层的厚度精度及成分准确性,是亟需解决的关键问题。
2019年美国桑迪亚国家实验室(Sandia National Laboratories,SNL)的Brown等[15]首次通过磁控溅射沉积工艺制备了一种新结构的Al-Cu梯度材料。该梯度材料由100个厚度为340 nm的周期调制层组成,每个周期层包含1个Al层和1个Cu层,通过调制每个周期层中Al层和Cu层的厚度,实现相邻周期层内同一材料物质的量比例1%的改变,从而在梯度材料34 μm的总厚度上,实现从纯Cu到纯Al的变化。由于Al层与Cu层没有混合,在较大程度上避免了金属间化合物的形成。经过检测,各层厚度与目标值间的差异在5%以内,平均峰谷值为(0.5±0.1) μm;光学轮廓测量显示平面度小于1 μm,因而,样品具有良好的平面性;X射线衍射(X-ray diffraction,XRD)结果显示,在Cu/Al界面处存在CuAl2,其含量小于0.1%。击靶实验与一维数值模拟结果显示,2次撞击Ta靶的实验中,初始应力分别为52和78 GPa,峰值应力分别为99和151 GPa,加载应变率都达到107 s−1。测得Ta在2次实验的应力和应变率条件下的强度为3~4 GPa。制备的Al-Cu体系的波阻抗范围较窄,可实现的应力与应变率调控范围较小。2021年印度巴巴原子研究中心(Bhabha Atomic Research Centre,BRAC)的Ray等[16]通过一维流体动力学计算发现,采用波阻抗范围更广的Mg-W体系时,周期调制梯度材料可达到不同的应力范围。美国的Brown等[15]发现, Al-Cu周期调制梯度材料在撞击5 μm厚Al靶的过程中,数值模拟的粒子速度曲线在斜波段并不呈平滑的加载波形,而是呈“振荡”式上升,但在43 μm厚的Ta靶实验中,数值模拟与实验都获得了平滑的加载波形。因此,靶材厚度的选取对于形成平滑的加载曲线至关重要。
基于文献研究结果,本研究将选择波阻抗差异更大、冶金反应简单的Ti(波阻抗2.2 g/(cm2∙μs))与Pt(波阻抗7.4 g/(cm2∙μs))体系,并采用更为高效的蒸镀方法制备Ti-Pt周期调制梯度材料,旨在实现更大的应力和应变率调控范围的准等熵加载,同时,将针对周期调制梯度材料加载波形的“振荡”上升现象开展数值模拟研究。
1. 实验与表征
1.1 Ti-Pt周期调制梯度材料的结构设计
在梯度材料斜波加载研究中,常采用波阻抗沿厚度方向变化的结构类型。波阻抗的定义为
Z=ρC (1) 波阻抗随厚度遵循以下关系[17]
Z(x)=Z0+A(xd)n (2) 式中:Z为材料波阻抗,Z0为低阻抗端的初始波阻抗,Z(x)为梯度材料沿厚度方向位置x处的波阻抗,ρ为材料密度,C为材料声速,A为比例系数,d为材料厚度,n为幂指数。通过改变幂指数n即可得到不同波阻抗分布类型的梯度材料。
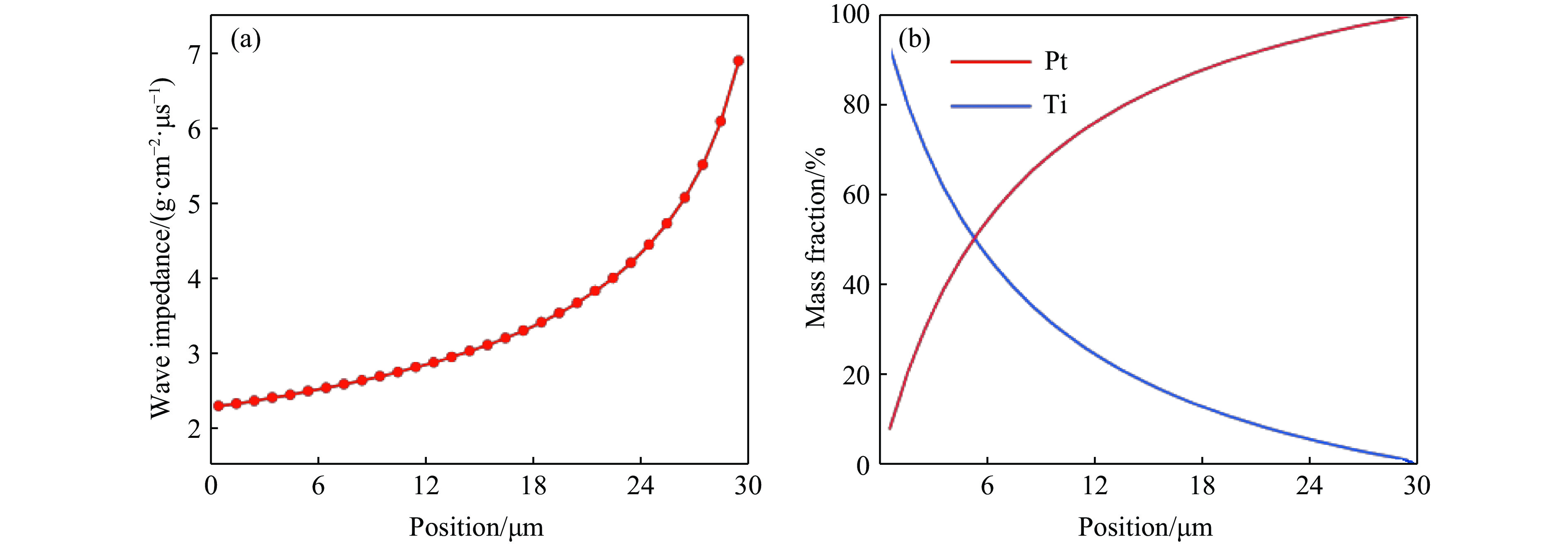
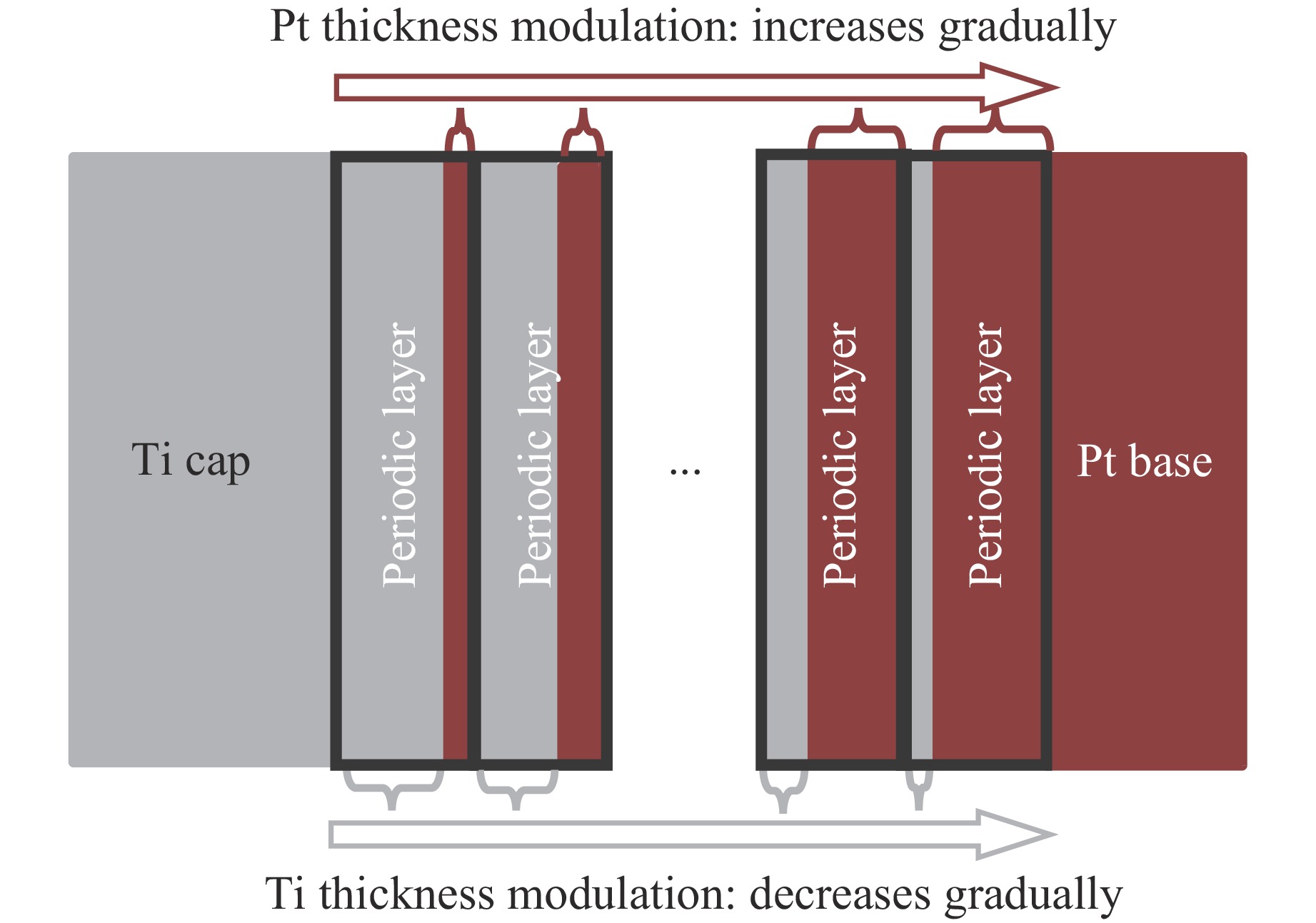
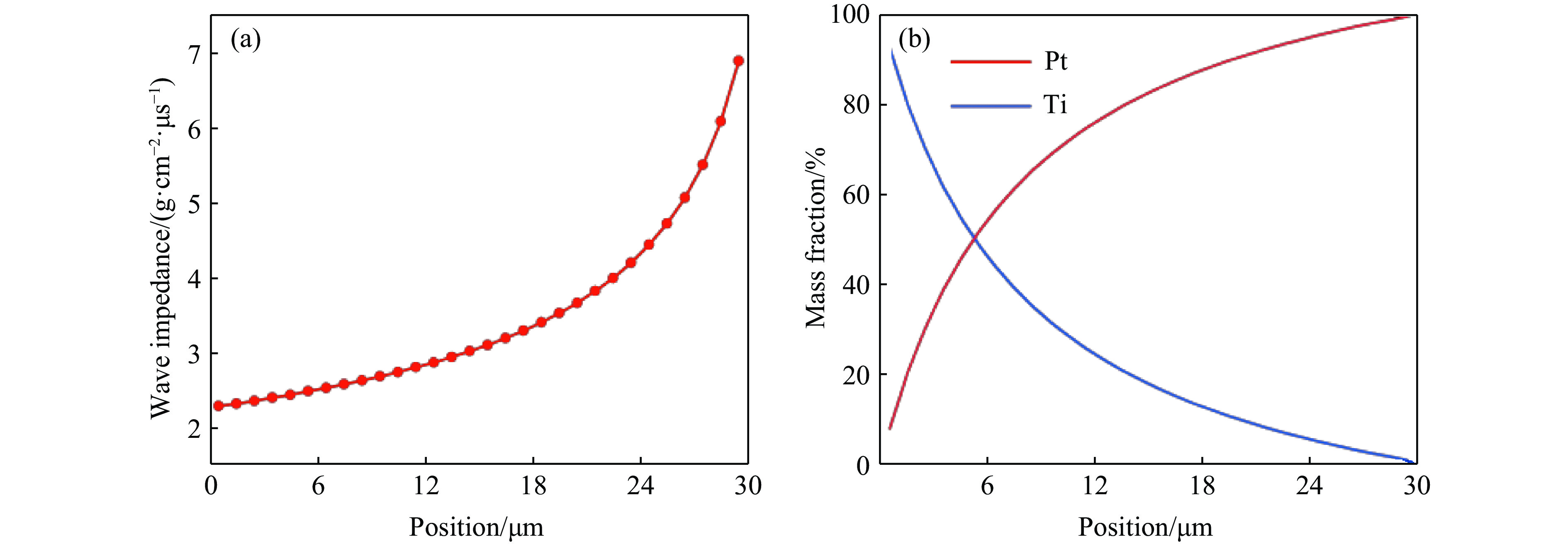
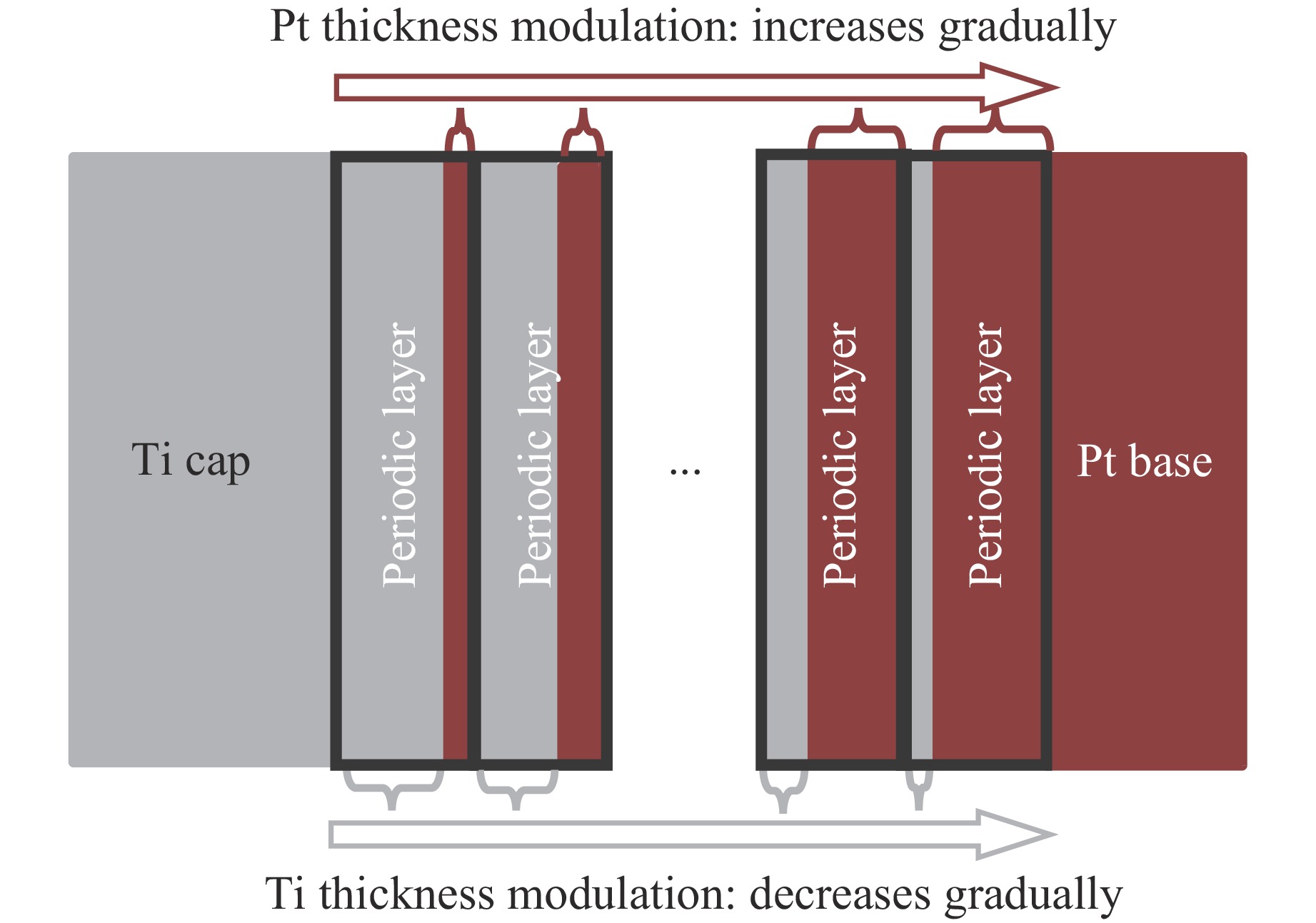
Ti-Pt周期调制梯度材料的结构如图1所示,从左向右,周期层中Ti层的厚度逐渐减小,Pt层的厚度逐渐增加。设计n=3的Ti-Pt周期调制梯度材料。幂指数越高,梯度材料对靶材的加载速率越低,而加载速率越低,表明由不可逆热耗散引起的热效应成分将会降低,进而使得压缩过程中熵增减小,准等熵压缩效果也相对较好。周期层厚度固定为1 μm,通过调制周期层内双组分(Ti和Pt单层)的厚度,实现波阻抗的梯度变化。周期层内Ti和Pt层的厚度遵循如下关系
Z(x)=ZTiHTiH+ZPtHPtH (3) HTi=HZPt−Z(x)ZPt−ZTi (4) HPt=HZ(x)−ZTiZPt−ZTi (5) 式中:H为周期层的厚度,HTi为周期层内Ti层的厚度,HPt为周期层内Pt层的厚度,ZTi为材料Ti的波阻抗,ZPt为材料Pt的波阻抗。
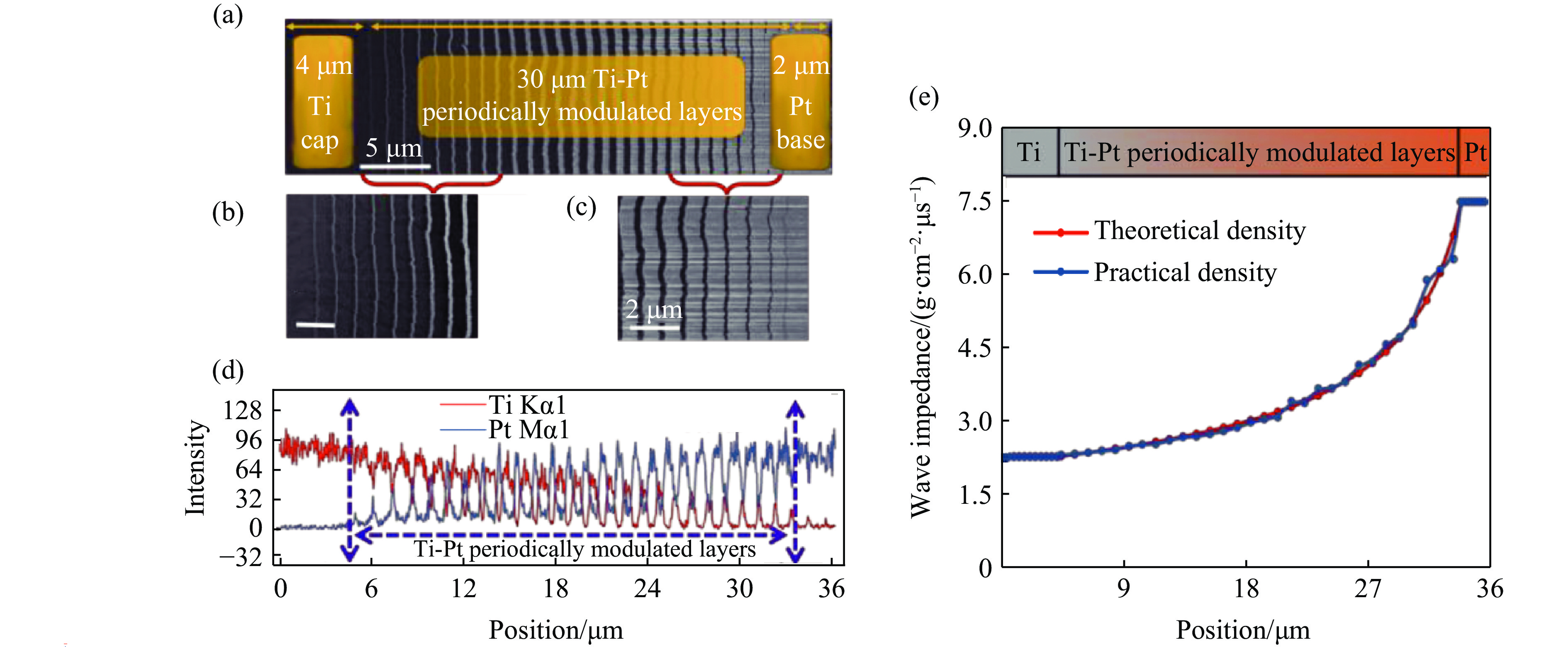
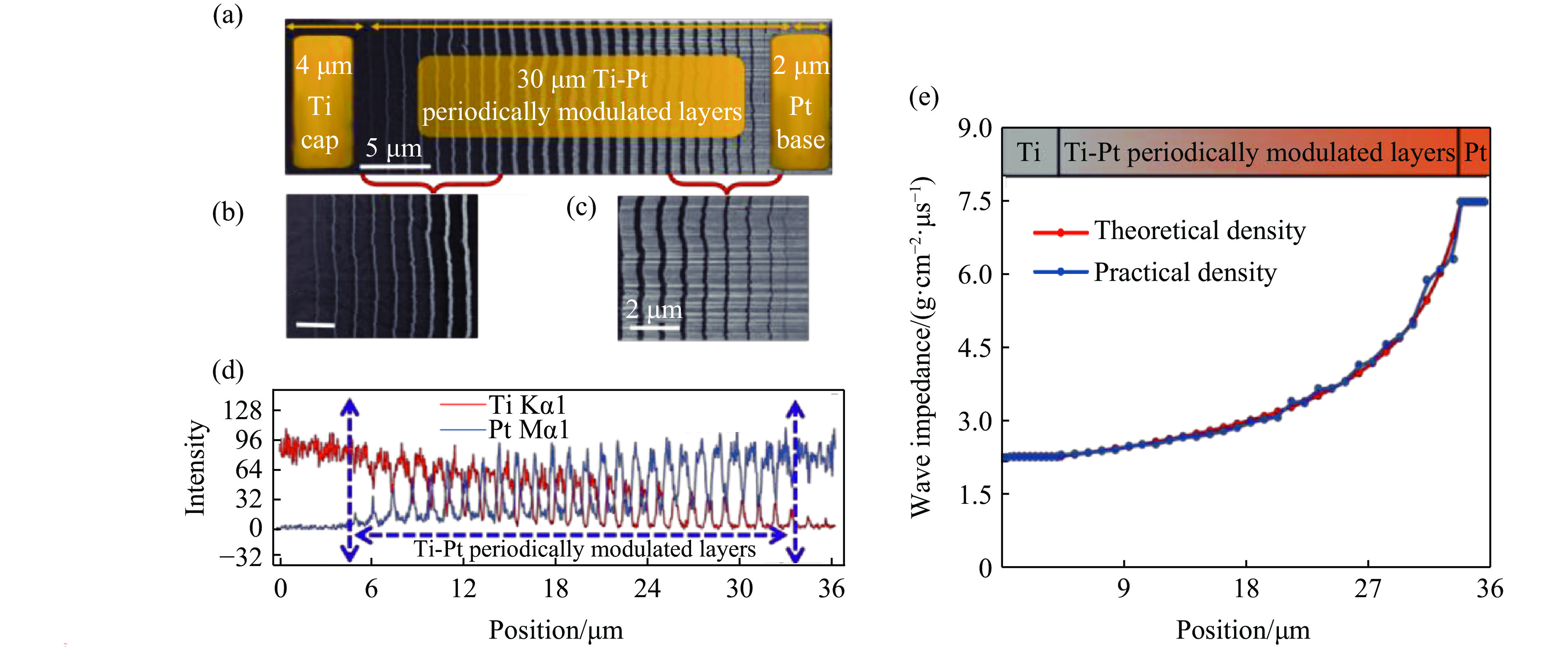
设计的Ti-Pt周期调制梯度材料的总厚度为36 μm(4 μm Ti帽、30 μm Ti-Pt周期调制层、2 μm Pt基底)。采用混合法则计算得到了30 μm Ti-Pt周期调制层内Ti、Pt的质量分数和波阻抗随厚度的变化关系,见图2。
1.2 Ti-Pt周期调制梯度材料的制备
1.2.1 原料及实验仪器
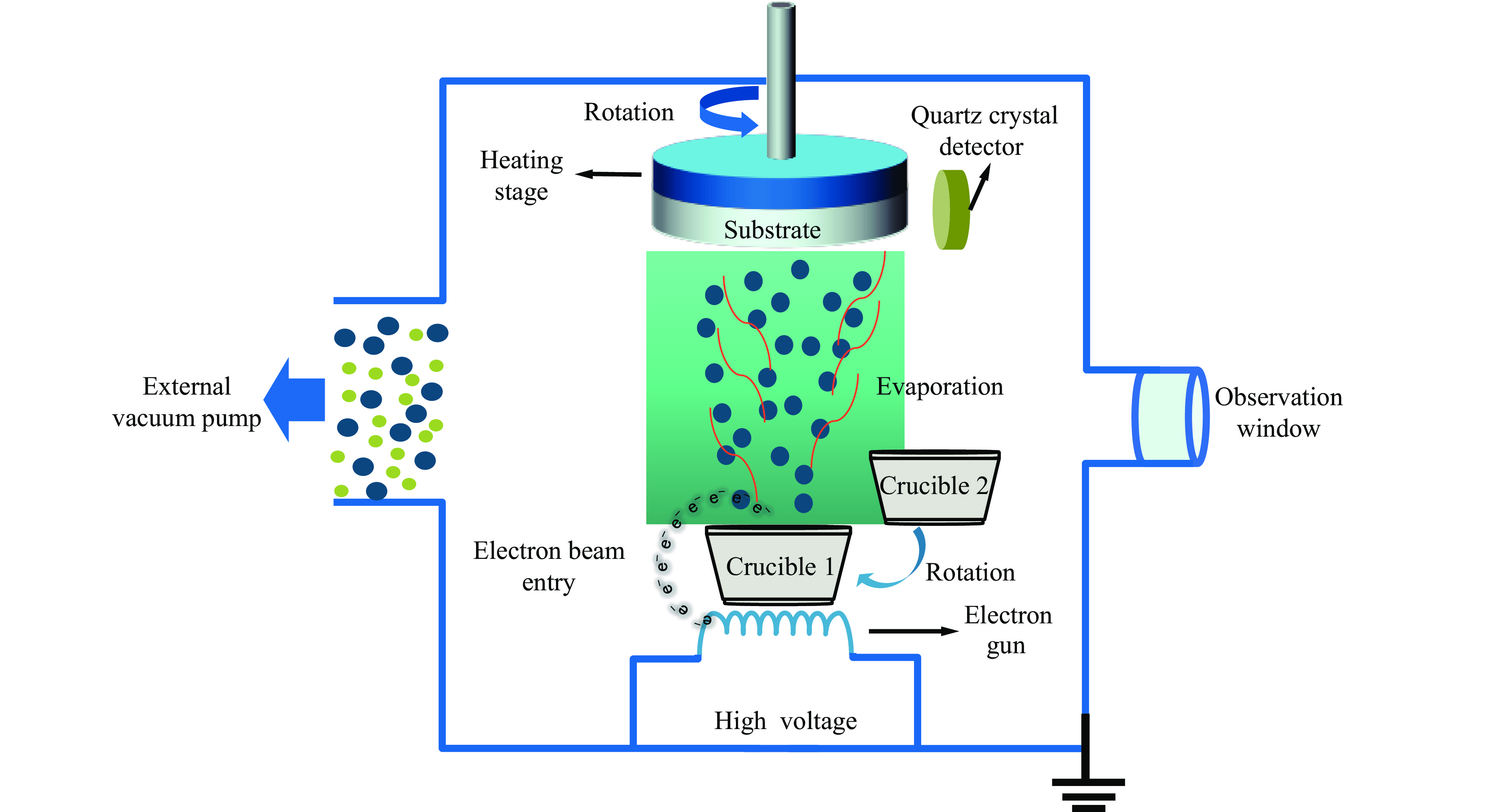
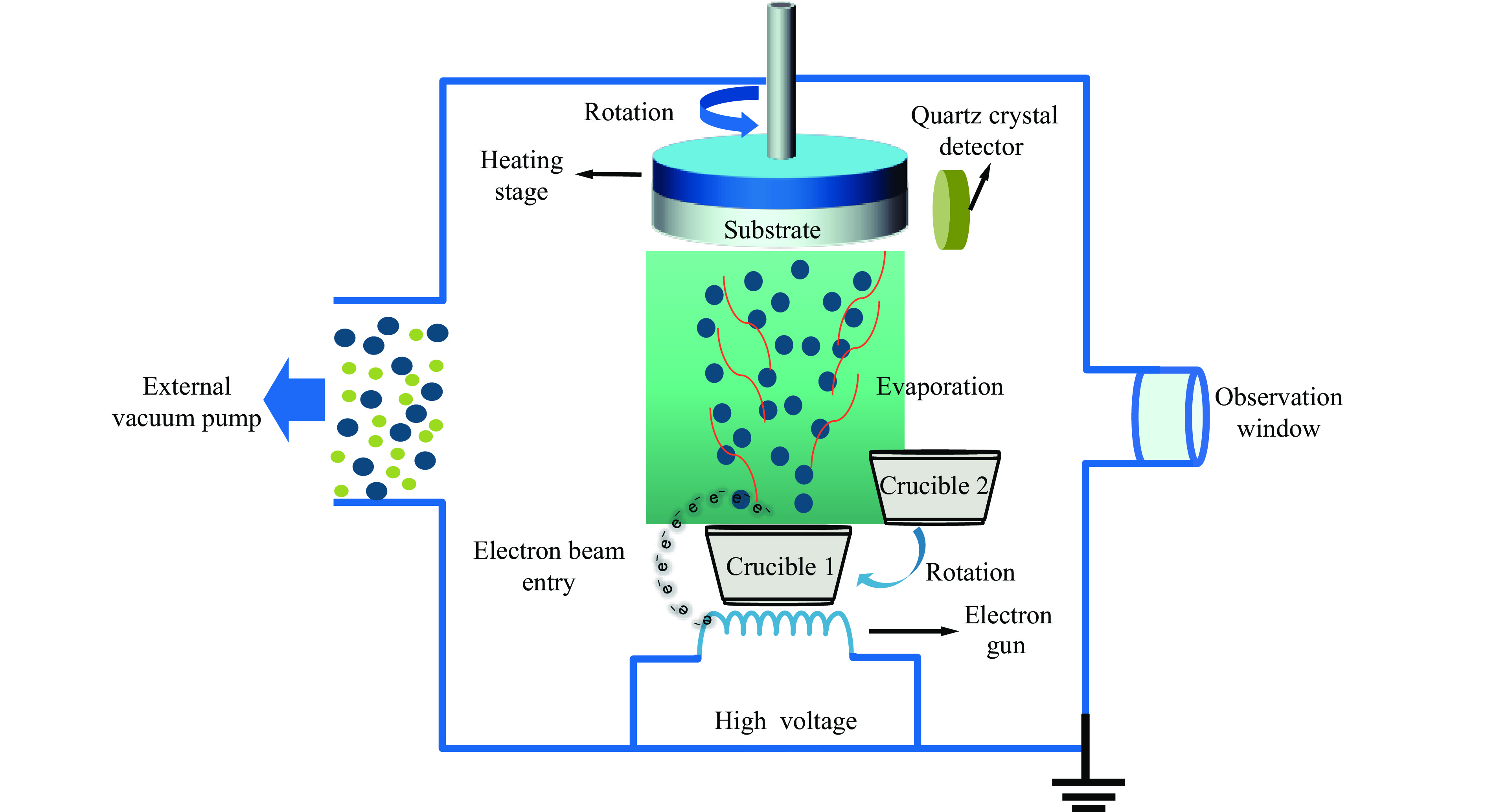
实验材料包括Ti圆片(∅3 mm×3 mm,纯度≥99.99%)、Pt圆片(∅5 mm×1 mm,纯度≥99.99%)、Al基片(∅13 mm×1 mm,纯度≥99.99%)、无水乙醇、丙酮;实验仪器包括超声波清洗仪器和电子束蒸发镀膜仪。电子束蒸发镀膜仪如图3所示,其基本原理是利用强高压在电子枪中激发出电子,电子在周围磁场的作用下形成定向电子束流轰击坩埚中的镀料,电子将动能转换为热能从而熔化镀料,镀料在真空环境中蒸发成气态原子,沉积至衬底形成镀层。
1.2.2 样品制备
抛光后的Al基片经无水乙醇和丙酮清洗后吹干,固定在可旋转的衬底上。将Pt与Ti镀料分别放置在不同的坩埚中。将腔室抽至6.0×10−4 Pa的真空度后,开始进行电子束蒸发沉积。通过坩埚的旋转实现Pt镀料与Ti镀料的交替沉积。Ti、Pt膜的沉积速率分别为2.5和1.0 Å/s,采用膜厚仪实时监控薄膜生长厚度。通过改变沉积时间控制薄膜的生长厚度。当一种材质的镀层生长到设计厚度后,及时切换坩埚以进行另一种材质的蒸镀。如此往复,通过60余次的切换,在Al基片上制备出周期数为30的Ti-Pt周期调制梯度材料。
1.3 Ti-Pt周期调制梯度材料的表征方法
采用探针式表面轮廓仪测量Ti-Pt周期调制梯度材料的厚度。通过扫描电子显微镜(scanning electron microscope,SEM)以及能谱分析仪(energy dispersive spectroscopy,EDS)观察和分析Ti-Pt周期调制梯度材料的断面(材料断面采用聚焦离子束制备)。采用XRD分析材料的物相及其结构,衍射源为Cu Kα,λ=
1.5418 Å,扫描速率为10°/min,扫描角度2θ为20°~80°。为评估材料的力学性能,采用纳米压痕仪器测试了材料3个不同位置处的硬度和弹性模量,每个量测试3次,结果取平均值,单次压入深度不超过材料总厚度的1/10,以免对基底产生影响,加载、保压及卸载时间分别为5、2和5 s,加载压力为50 mN。1.4 Ti-Pt周期调制梯度材料的准等熵加载实验
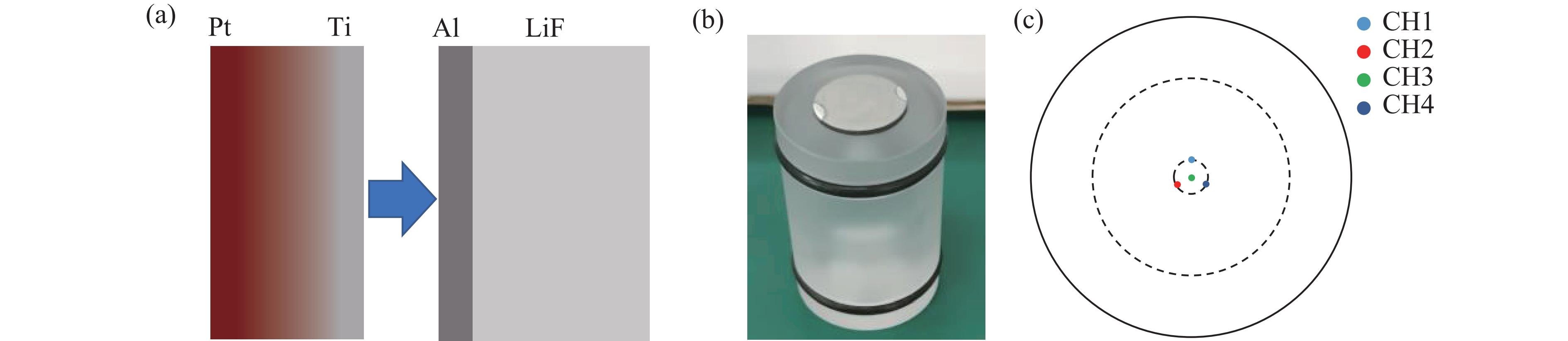
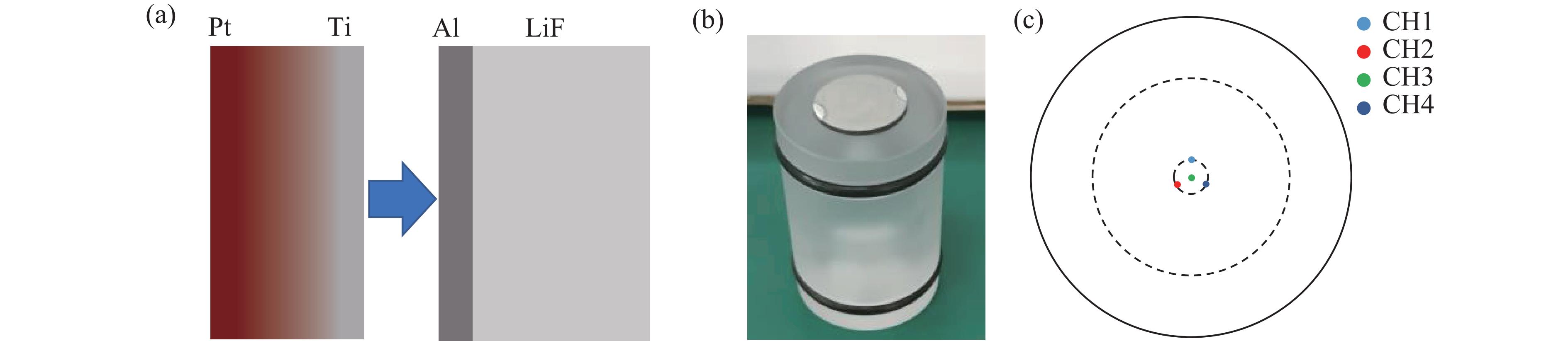
实验采用的发射装置为一级轻气炮,其构造主要包括高压气室、发射管和靶室3部分。高压气室与抽真空系统及高压注气系统相连。在实验准备完成后,首先,使用抽真空装置使整个系统处于指定的真空度;然后,采用高压注气系统向气室内注入气体(通常是氮气),直至达到所需压力。将梯度材料粘结在弹托前端,弹托发射时,打开气室的电磁发射阀,气室内的高压气体直接作用于弹托底部,装载梯度材料的弹托加速通过发射管进入靶室并撞击样品[18–19]。弹托的直径为23.9 mm,弹托前端粘结直径为13 mm的Ti-Pt周期调制梯度材料。第1发轻气炮实验以480.9 m/s的加载速度发射装有Ti-Pt周期调制梯度材料的弹托撞击镀有5 μm铝膜的LiF窗口;第2发轻气炮实验中,以541.6 m/s的加载速度发射Ti-Pt周期调制梯度材料撞击镀有60 μm厚铝膜的LiF窗口。图4(a)为Ti-Pt周期调制梯度材料撞击Al靶材的示意图,图4(b)为粘结Ti-Pt周期调制梯度材料的弹托,图4(c)为LiF窗口背面的光纤测速点布局。采用全光纤激光干涉测速仪(displacement interferometer system for any reflector,DISAR)探测Al/LiF界面的粒子速度。
 图 4 Ti-Pt周期调制梯度材料的准等熵加载实验设计:(a) Ti-Pt周期调制梯度材料撞击Al靶示意图,(b) 粘结Ti-Pt周期调制梯度材料的弹托,(c) LiF窗口背面分布的光纤测速点Figure 4. Experimental design of Ti-Pt periodically modulated gradient material: (a) schematic diagram of Ti-Pt periodically modulated gradient material impacting Al target; (b) a projectile bonded with Ti-Pt periodically modulated gradient material; (c) the distribution of velocity measurement fiber on the back of LiF window
图 4 Ti-Pt周期调制梯度材料的准等熵加载实验设计:(a) Ti-Pt周期调制梯度材料撞击Al靶示意图,(b) 粘结Ti-Pt周期调制梯度材料的弹托,(c) LiF窗口背面分布的光纤测速点Figure 4. Experimental design of Ti-Pt periodically modulated gradient material: (a) schematic diagram of Ti-Pt periodically modulated gradient material impacting Al target; (b) a projectile bonded with Ti-Pt periodically modulated gradient material; (c) the distribution of velocity measurement fiber on the back of LiF window1.5 Ti-Pt周期调制梯度材料的准等熵加载数值模拟
采用一维弹塑性拉格朗日流体动力学方程组[20]描述Ti-Pt周期调制梯度材料的冲击加载或准等熵加载过程。本研究中Ti-Pt周期调制梯度材料加载过程的模拟主要基于中国工程物理研究院流体物理研究所自主开发的多介质弹塑性拉格朗日流体动力学计算程序,其运动方程、连续性方程和能量方程为
{˙u=−Rα−1∂σR∂m−(α−1)vR(σR−σθ)v=Rα−1∂R∂m˙E=−(p+q)˙v+v[S1˙ε1+(α−1)S2˙ε2] (6) 式中:R为欧拉坐标系内的径向坐标,u为粒子速度,˙R=u,˙u为随体微商,α=1,2,3分别对应平面问题、柱面问题和球面问题,σR为径向应力,σθ为环向应力,m为具有质量量纲的拉氏变量(α=1时为质量),v为比容,q为人工黏性,S1、S2为应力偏量,˙ε1、˙ε2为应变率,p为压力,E为比内能。
Ti-Pt周期调制梯度材料及靶材的相关物性参数列于表1,其中:ρ0为密度,C0为体积声速,λ为雨贡纽系数,γ为格林内森系数,cV为比定容热容。通过式(3)~式(5)建立Ti-Pt周期调制梯度材料的数值模型,Ti层与Pt层的网格边长均设置为10 nm,其他材料的网格尺寸保持与Ti和Pt层一致(10 nm),以确保模拟过程的准确性。
2. 结果与讨论
2.1 Ti-Pt周期调制梯度材料的厚度
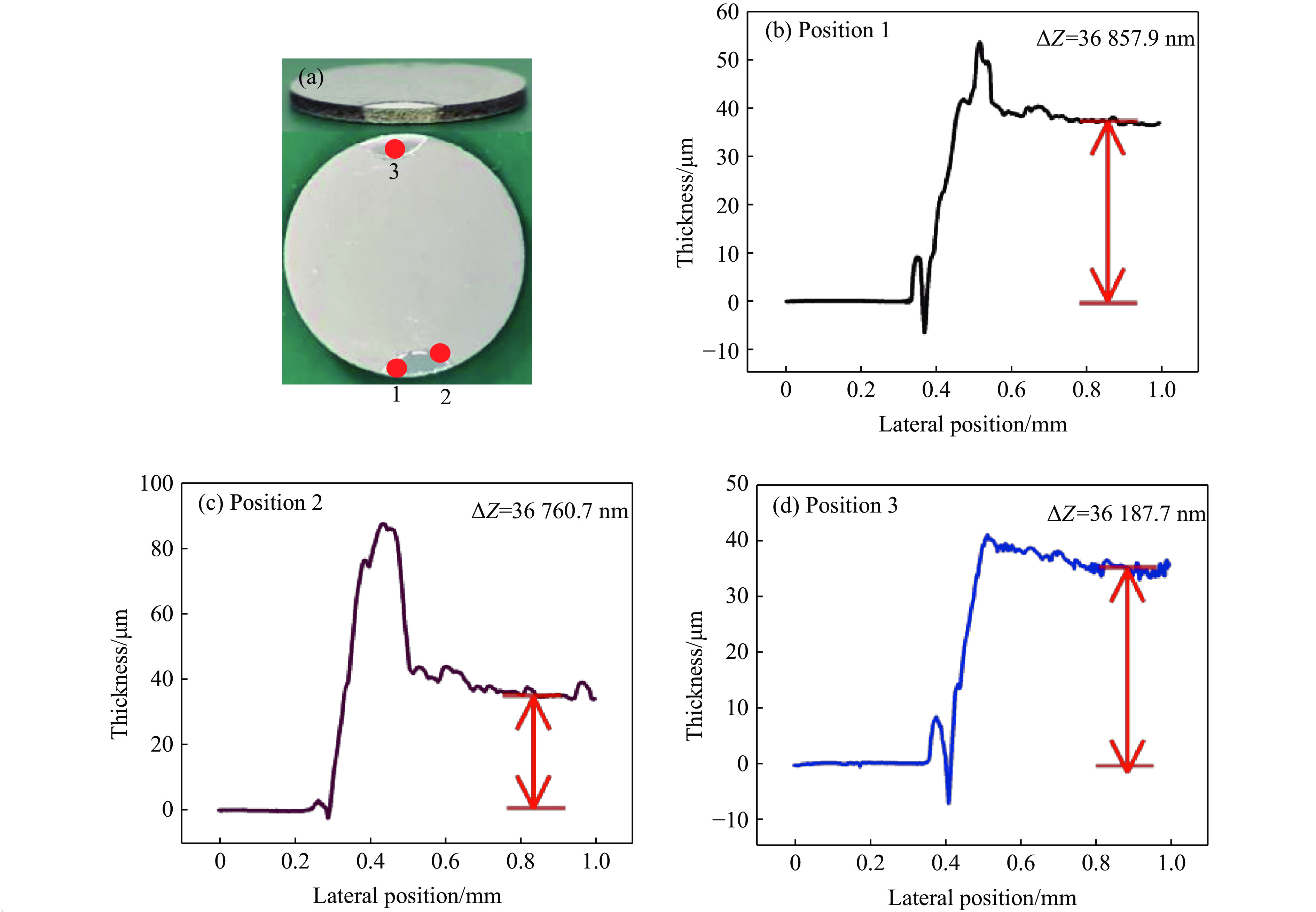
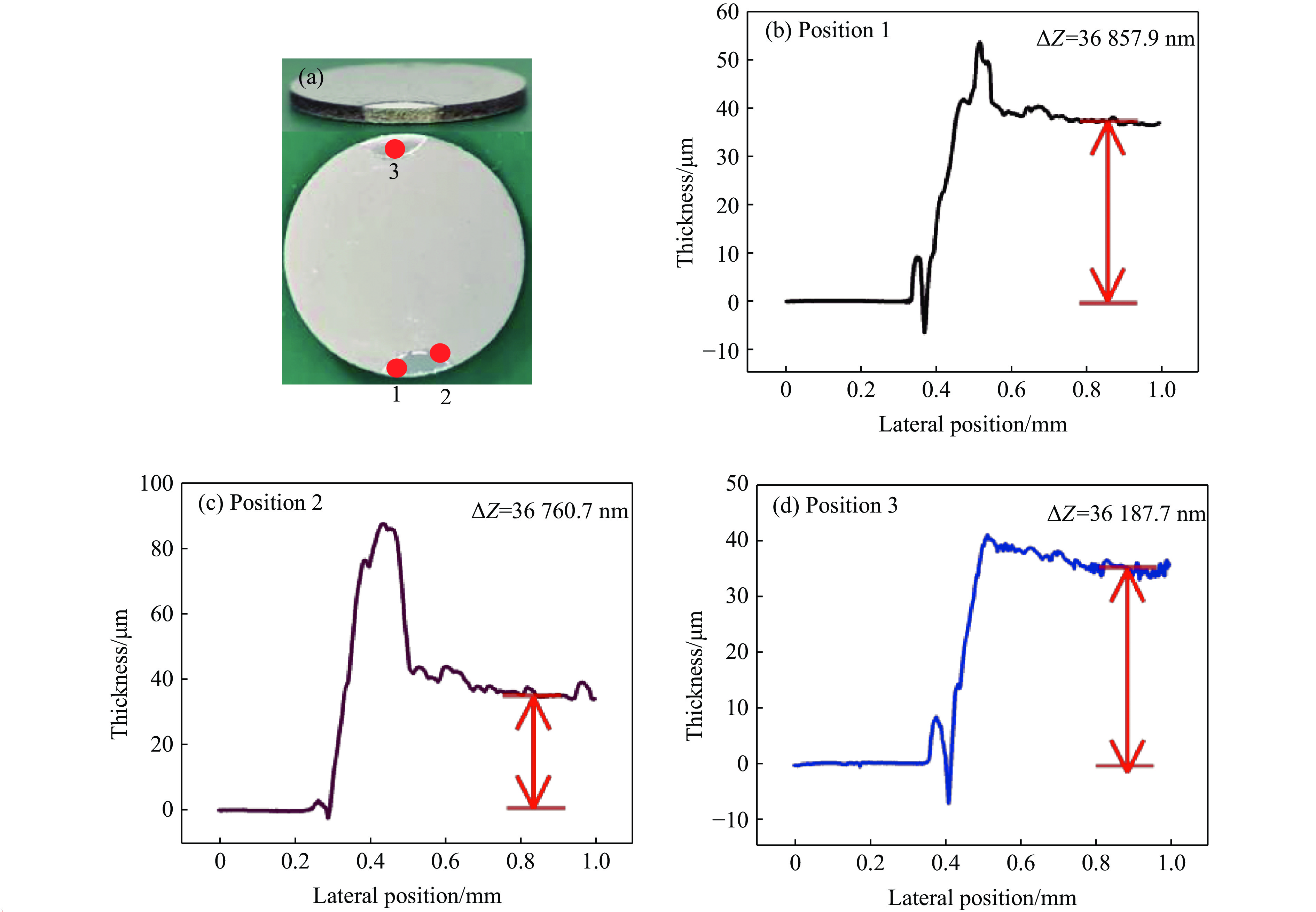
图5(a)为Ti-Pt周期调制梯度材料样品,可以看出梯度材料表面平整且层间平行度较好,边缘两处凹陷区是由夹具夹持造成的。图5(b)~图5(d)分别为样品3个不同位置处测得的厚度,测量结果表明,实际厚度的误差处于0.1~0.9 μm之间,3处位置的平均厚度为
36602.1 nm,与理论值的偏差为1.67%,说明实际厚度与设计值符合较好。2.2 Ti-Pt周期调制梯度材料截面形貌
图6(a)为Ti-Pt周期调制梯度材料截面的SEM整体图像,图6(b)和图6(c)分别为富Ti区与富Pt区的SEM图像,其中:浅色为Pt层,深色为Ti层。可以看出,Pt与Ti的层间界面清晰,双组分过渡连续且结合紧密,制备的样品实现了从富Ti区到富Pt区的渐变。图6(d)为EDS线扫描结果,可以看出,Pt与Ti成分在30个周期层中均实现了交替排列。Ti-Pt周期调制梯度材料的波阻抗随材料位置的分布曲线如图6(e)所示,其中:红线为理论设计曲线,蓝线为实测波阻抗分布曲线。实测波阻抗分布曲线是通过SEM图像获得的每层厚度结合式(3)得到的。结果显示,不同周期调制层的波阻抗最大误差为6.2%,平均误差为2.4%,理论设计的波阻抗与实测结果吻合较好,宏观上实现了波阻抗从纯Ti到纯Pt的过渡。
 图 6 Ti-Pt周期调制梯度材料的截面形貌(a),富Ti区的形貌(b),富Pt区的截面形貌(c), 能谱分析图(d),理论与实际波阻抗随位置的分布曲线(e)Figure 6. Overall view (a); Ti-rich region view (b); Pt-rich region view (c); energy spectrum analysis diagram (d); theoretical and practical wave impedance distribution curve with position (e) for the Ti-Pt periodically modulated gradient material
图 6 Ti-Pt周期调制梯度材料的截面形貌(a),富Ti区的形貌(b),富Pt区的截面形貌(c), 能谱分析图(d),理论与实际波阻抗随位置的分布曲线(e)Figure 6. Overall view (a); Ti-rich region view (b); Pt-rich region view (c); energy spectrum analysis diagram (d); theoretical and practical wave impedance distribution curve with position (e) for the Ti-Pt periodically modulated gradient material2.3 Ti-Pt周期调制梯度材料的物相分析
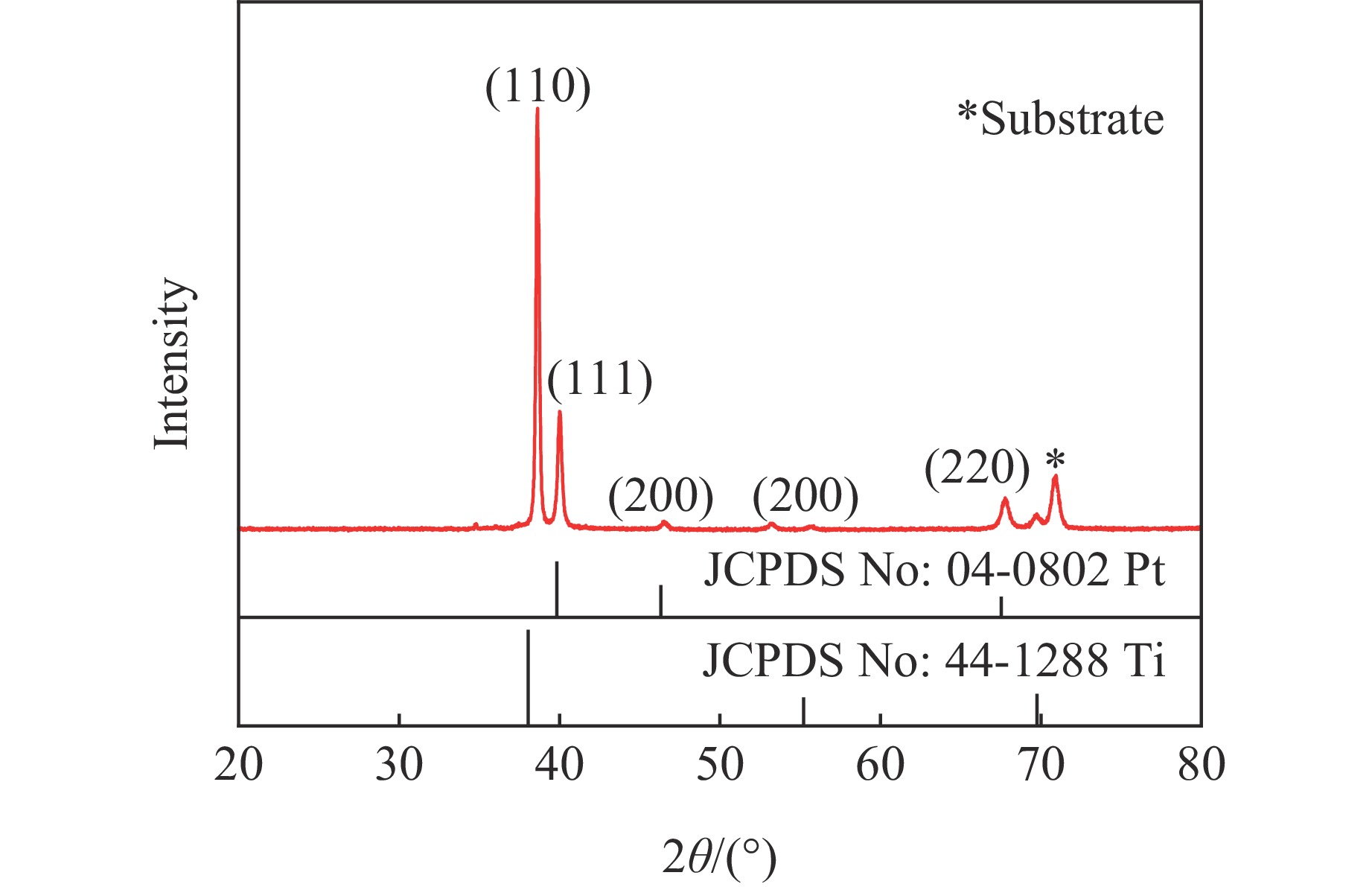
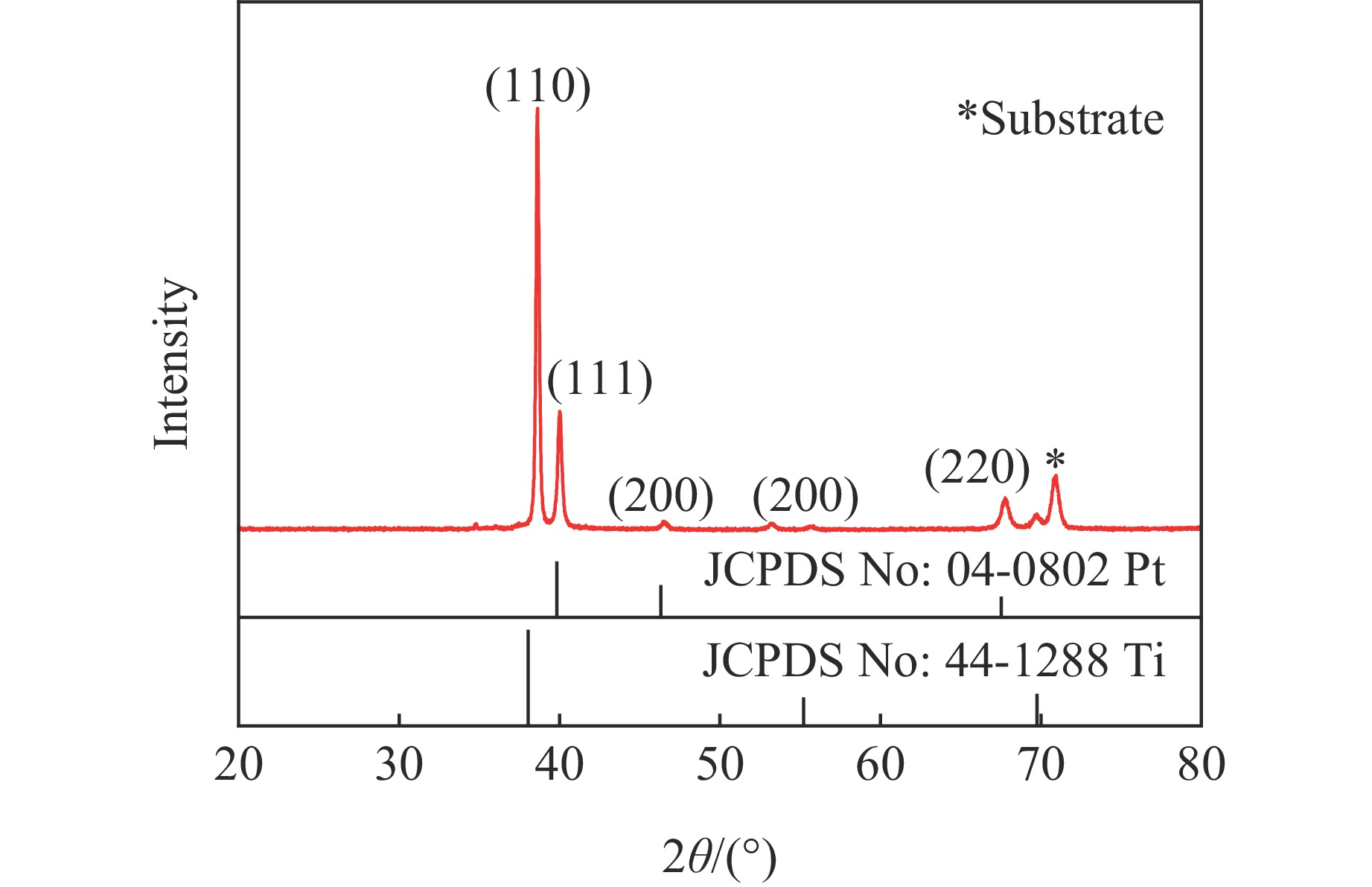
Ti-Pt周期调制梯度材料的XRD谱如图7所示。图7表明,所制备材料由单相Ti和Pt组成,未发现Ti-Pt合金相,说明界面处没有发生明显的扩散及冶金反应。
2.4 Ti-Pt周期调制梯度材料的力学性能
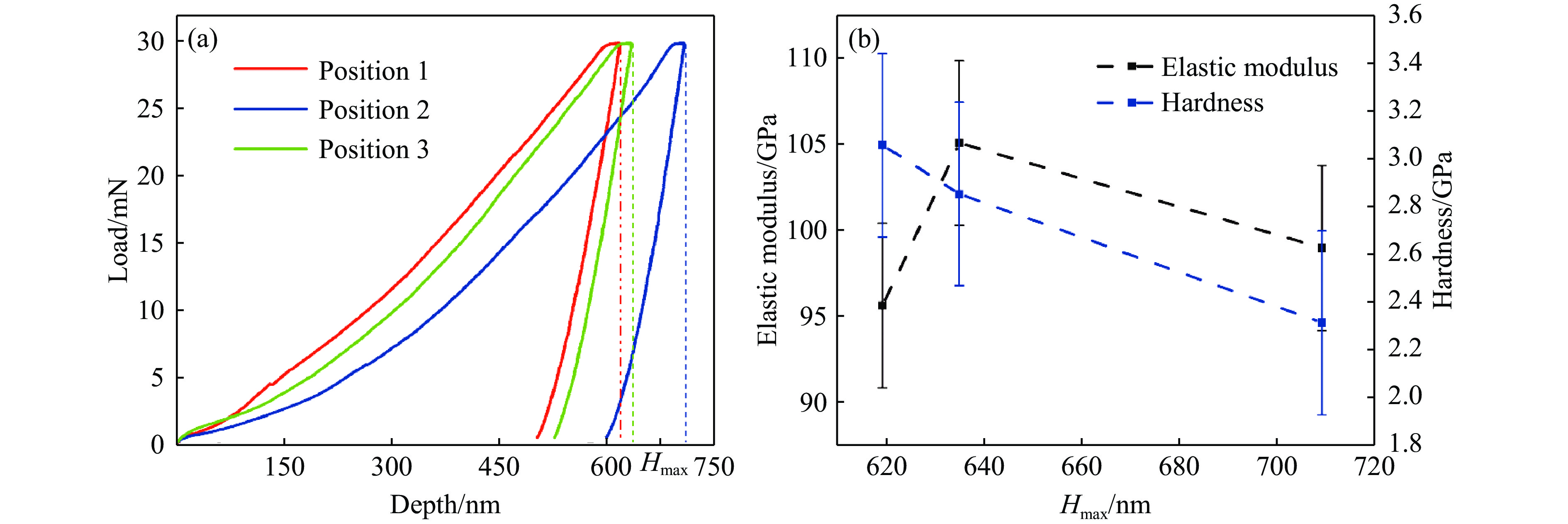
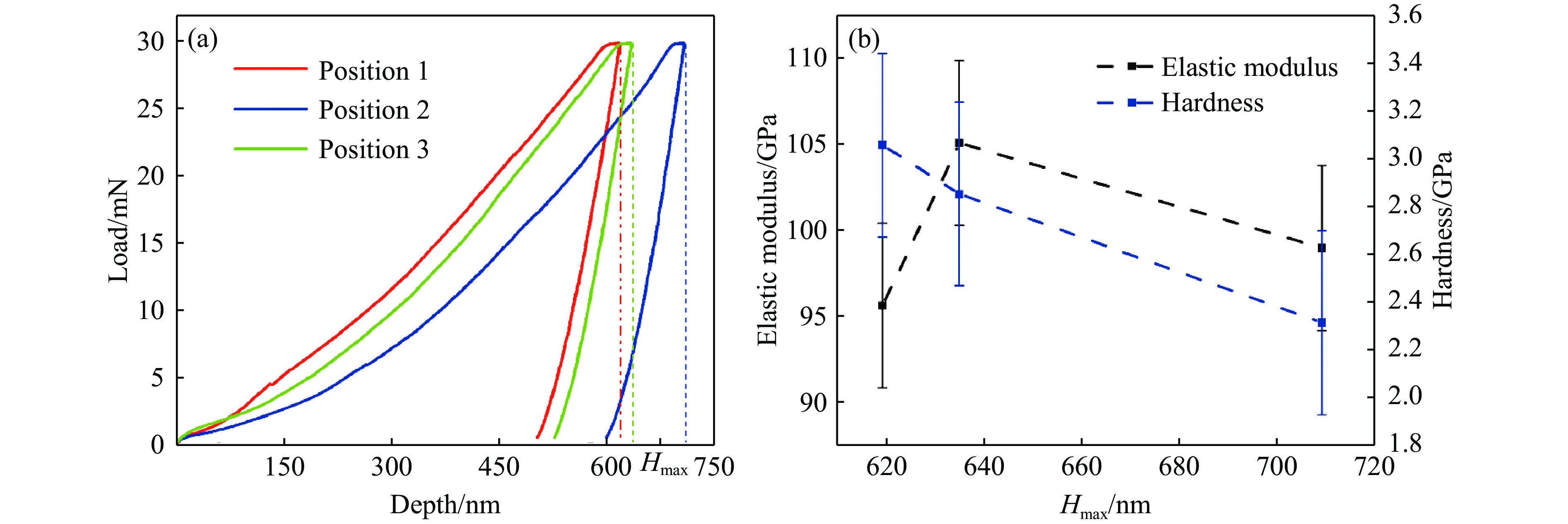
在轻气炮的高速加载下,梯度材料会承受一定的压力。为了确保梯度材料在撞击靶材时保持完整,其必须具备有一定的力学性能,以抵抗高速飞行时的压力。为此,需开展Ti-Pt周期调制梯度材料的纳米压痕测试。在3个不同位置开展相同压力下的压入测试,测试结果如图8所示。图8(a)为3个位置处的加-卸载曲线,可以看出,最大压入深度为710 nm,最小压入深度为620 nm,3次压入深度均低于Ti-Pt周期调制梯度材料总厚度的1/10,因而,压入对基底无影响。同时,加-卸载结果还表明,不同位置处加载曲线的重叠性较好。图8(b)为3个位置处的弹性模量及硬度,结果表明:最大弹性模量为105.1 GPa,最小弹性模量为95.6 GPa,平均弹性模量为99.8 GPa;最大硬度为3.1 GPa,最小硬度为2.3 GPa,平均硬度为2.8 GPa。通常来说,粉末冶金方法制备的Al-Cu梯度材料界面处的最大硬度仅为0.6 GPa,所以,制备的Ti-Pt周期调制梯度材料具有较为优异的力学强度,有利于提升其发射过程中的完整性。
2.5 Ti-Pt周期调制梯度材料的加载特性
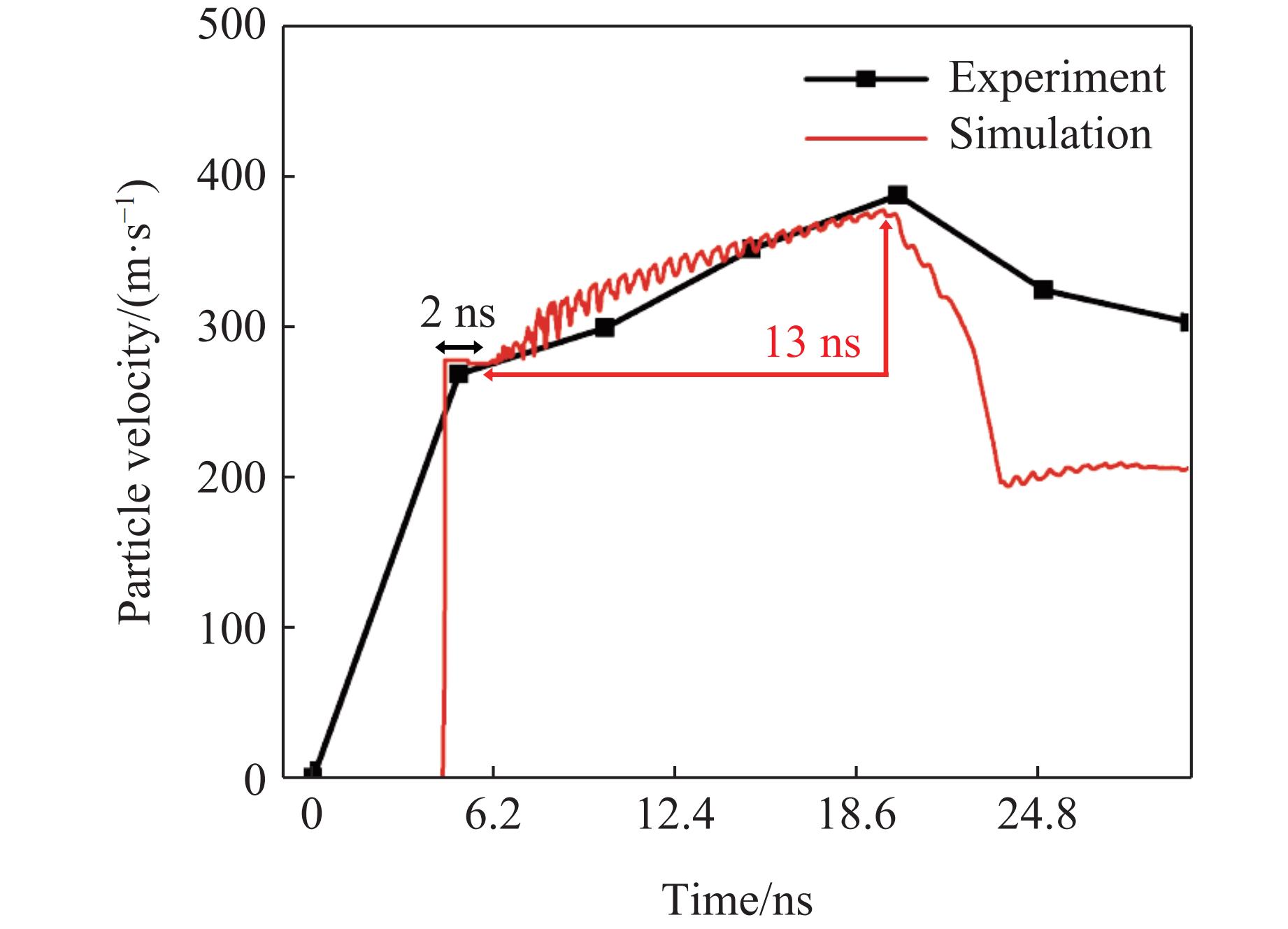
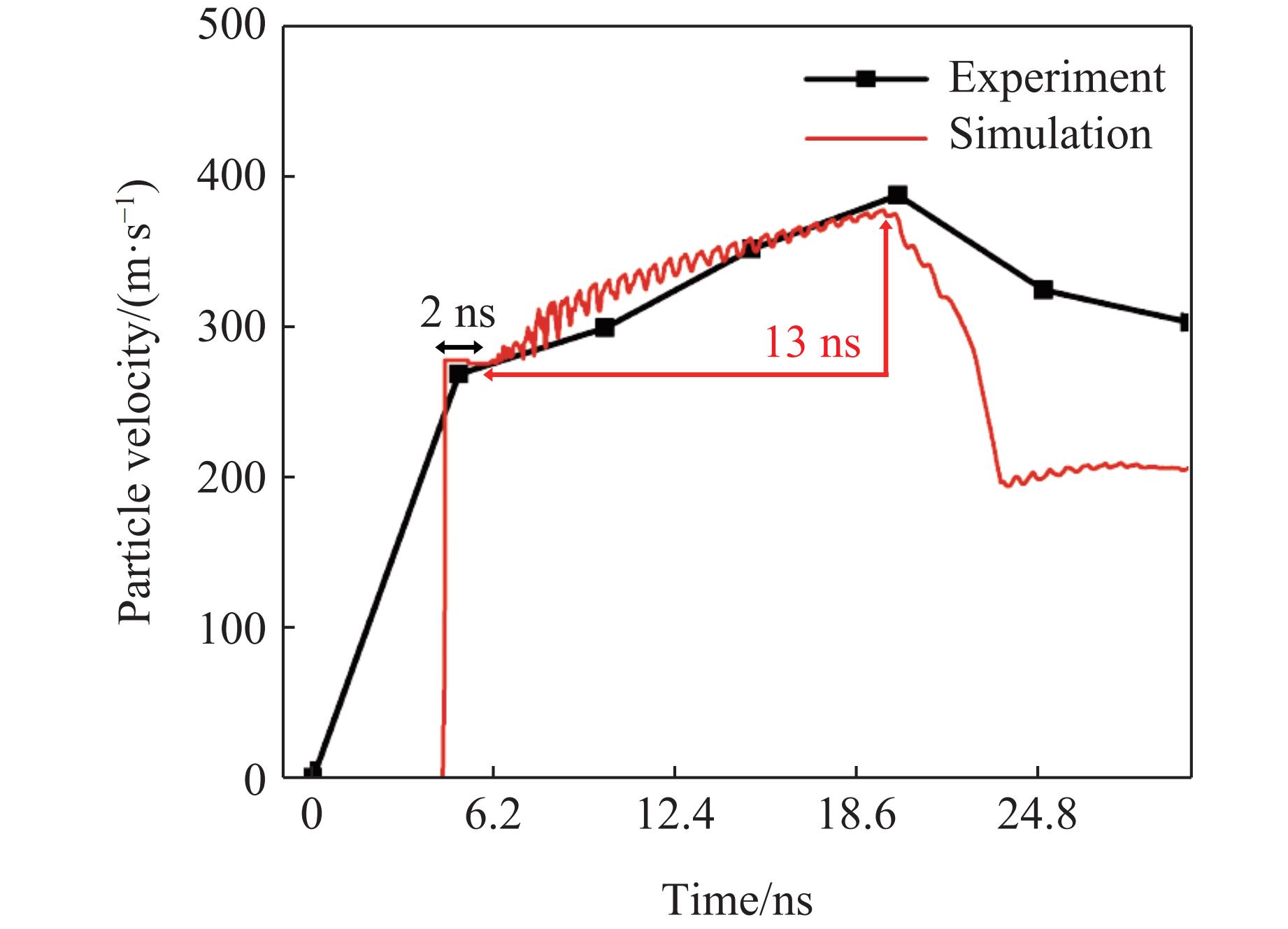
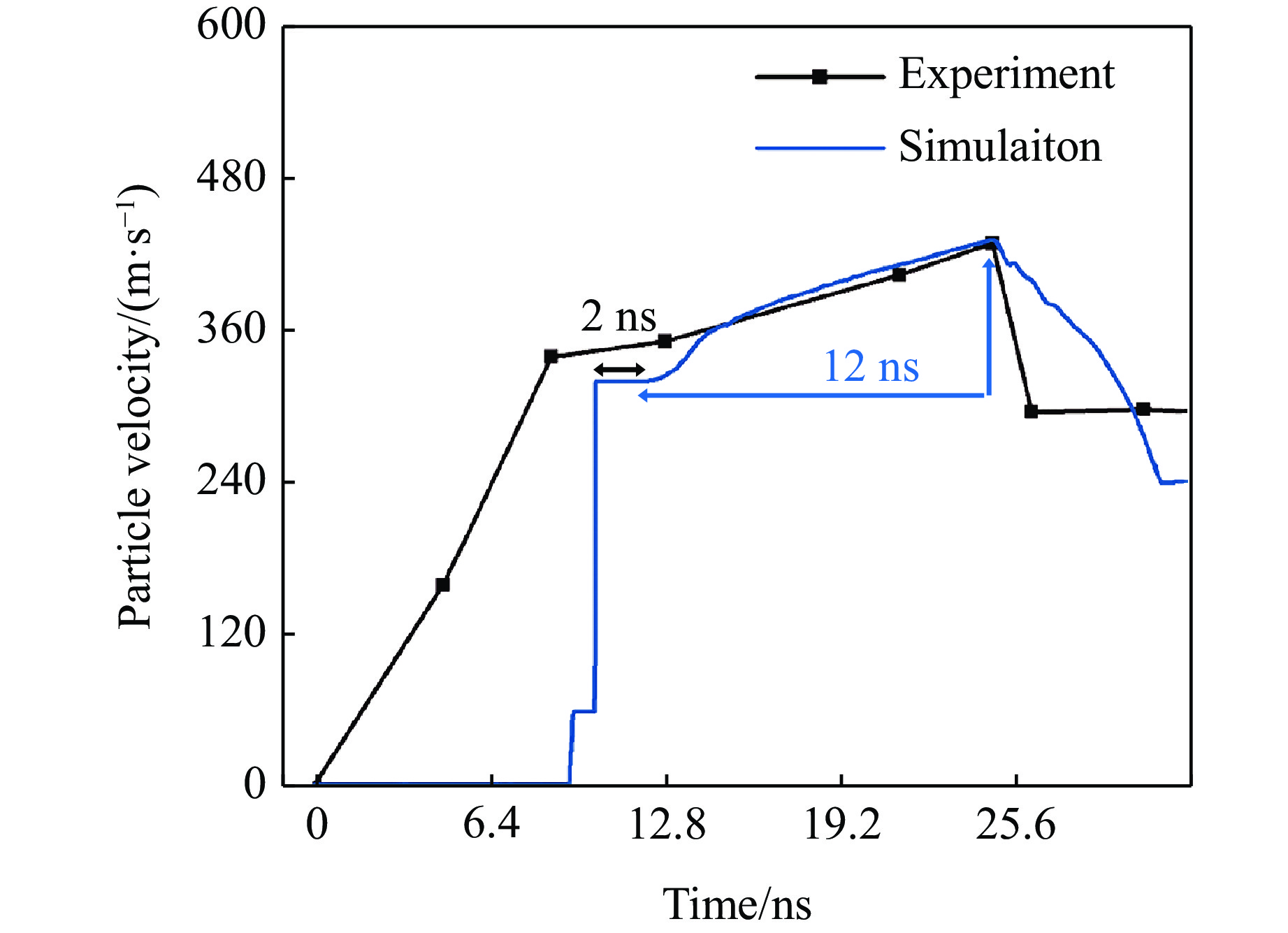
图9显示了Ti-Pt周期调制梯度材料以480.9 m/s的速度撞击5 μm厚Al靶的轻气炮加载实验与数值模拟结果,其中:黑线圆点为实验获得的粒子速度,红线为数值模拟结果。结果表明,36 μm厚的Ti-Pt周期调制梯度材料成功实现了准等熵加载,初始冲击速度平台持续2 ns左右,准等熵加载段持续13 ns左右,模拟结果与实验吻合良好。
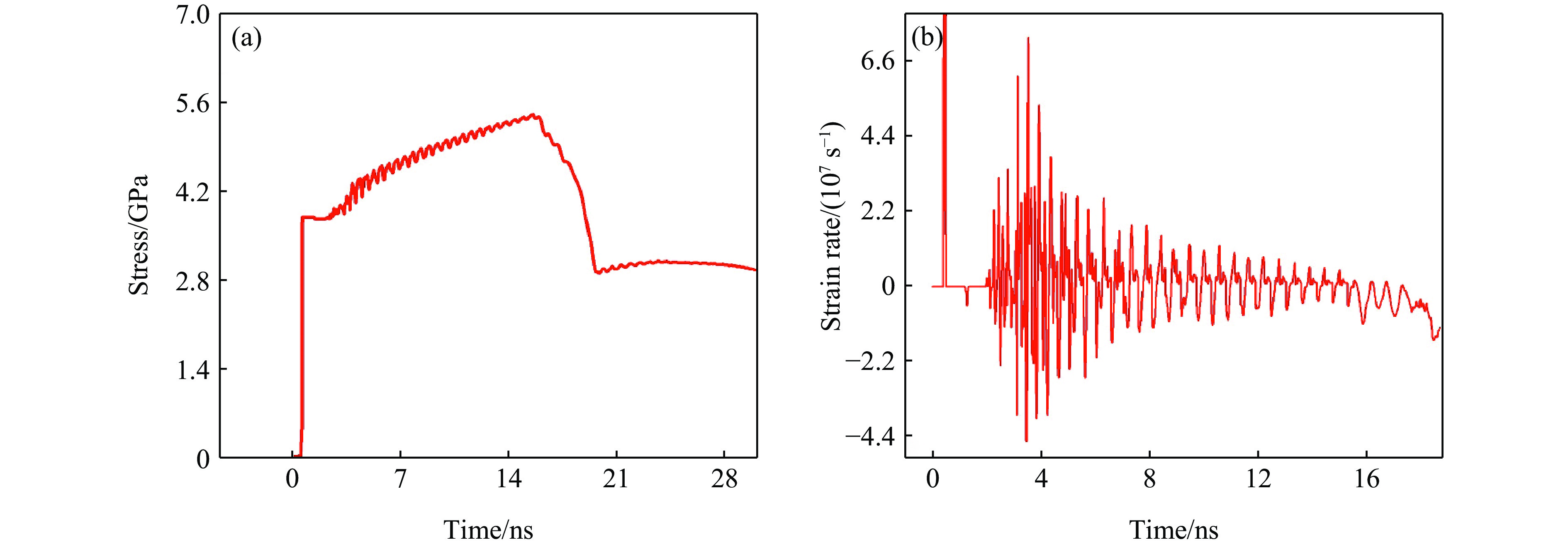
图10为数值模拟获得的Ti-Pt周期调制梯度材料撞击5 μm 厚Al靶时产生的应力及应变率与时间关系。结果表明,以480.9 m/s速度撞击Al靶时,应力从初始的4.0 GPa连续上升至峰值应力5.5 GPa(图10(a));应变率曲线在正负值间持续振荡,并且幅度较大,最大应变率为7.3×107 s−1,最小应变率为−4.5×107 s−1,平均应变率为1.3×106 s−1(图10(b))。
值得注意的是,图9中实验粒子速度曲线表明其为平滑的准等熵加载,而图9中的数值模拟曲线以及图10中的应力、应变率曲线在准等熵加载段的振荡幅度较大,其原因是实验受限于示波器采样率、带宽等硬件条件,在13 ns时间量级内,能采集到的有效数据点较少,波形曲线只会展现出加载上升趋势。数值模拟结果则不仅在曲线上升趋势上与实验结果吻合良好,而且包含更多击靶过程中的加载细节。
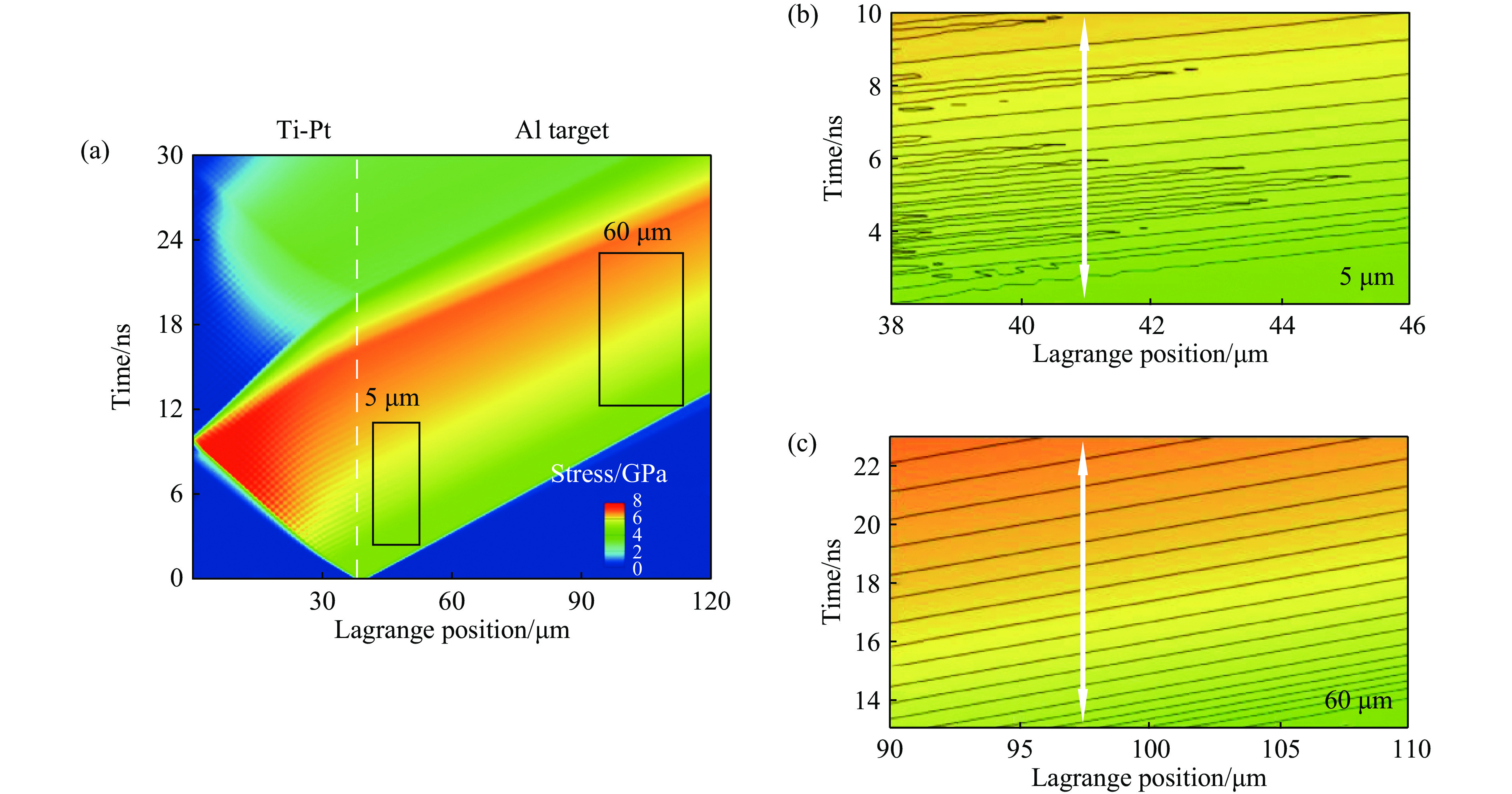
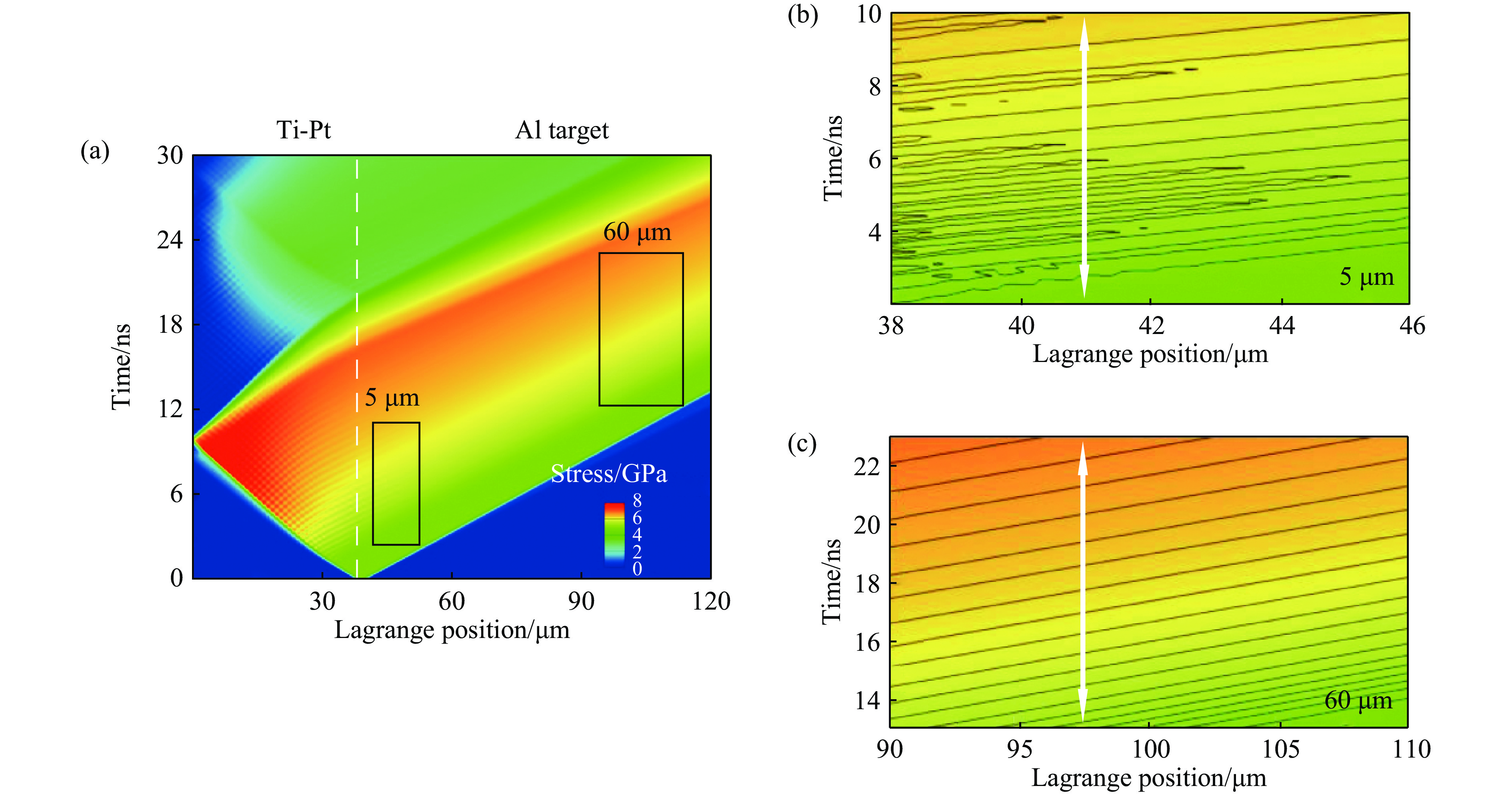
通过数值模拟应力云图对曲线“振荡”原因进行分析。在其他参数不变的条件下,将Al靶厚度从5 μm增大至80 μm,重新进行数值模拟,观察Ti-Pt周期调制梯度材料的波系整合过程,结果如图11所示。图11(a)所示的应力云图显示,2个位置处的应力均从初始的4.0 GPa上升至峰值5.5 GPa。在局部应力云图中(图11(b)和图11(c)),黑色实线为应力等值线,等值线间隔为0.1 GPa。在图11(b)中,当靶材厚度为5 μm时,准等熵段多道等值线交汇在一起,表明该位置的应力并不是单调上升,在压缩波与稀疏波的共同作用下呈先上升后下降再上升的趋势,因而,应力曲线呈现“振荡”现象。当靶材增厚至60 μm时,等值线在图11(c)中呈现平行排列的上升形式,代表该处多个波系完成了整合,靶材持续受压缩波作用,应力曲线呈现平滑的加载波形。应力云图结果表明,Ti-Pt周期调制梯度材料在撞击靶材后会产生多个波系的追赶、叠加以及整合,30周期层的Ti-Pt周期调制梯度材料在5 μm厚的Al靶材中无法完成波系整合,因而无法形成平滑的准等熵加载过程,当Al靶厚度增大至60 μm时,可以实现良好的准等熵加载效果。
 图 11 Ti-Pt周期调制梯度材料撞击Al靶时的应力云图:(a) 0~120 μm区间内的应力云图,(b) 38~47 μm区间内的应力细节云图,(c) 90~110 μm区间内的应力云图Figure 11. Stress contour map during Ti-Pt periodically modulated gradient material impacting Al target: (a) stress contour map from 0 to 120 μm; (b) detailed stress contour map from 38 μm to 47 μm; (c) detailed stress contour map from 90 μm to 110 μm
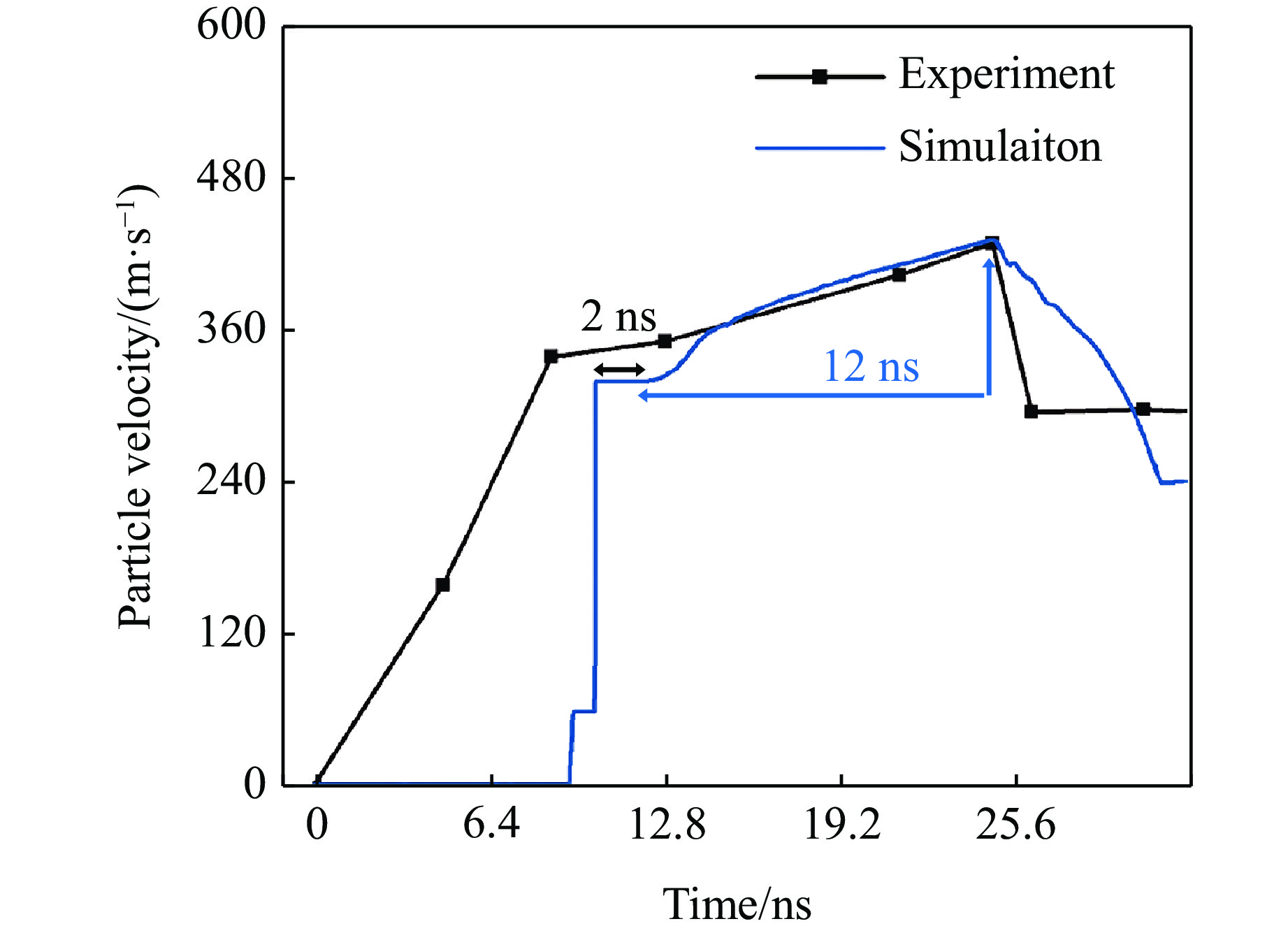
图 11 Ti-Pt周期调制梯度材料撞击Al靶时的应力云图:(a) 0~120 μm区间内的应力云图,(b) 38~47 μm区间内的应力细节云图,(c) 90~110 μm区间内的应力云图Figure 11. Stress contour map during Ti-Pt periodically modulated gradient material impacting Al target: (a) stress contour map from 0 to 120 μm; (b) detailed stress contour map from 38 μm to 47 μm; (c) detailed stress contour map from 90 μm to 110 μm将Al靶厚度增大至60 μm,重新开展轻气炮加载实验。Ti-Pt周期调制梯度材料以541.6 m/s的速度撞击60 μm厚Al靶的轻气炮加载实验与数值模拟结果(如图12所示)。受实验硬件等条件的影响,2次气炮实验的发射速度略有差异(此次轻气炮实验的发射速度相较于前一次实验提高了60.7 m/s),但处于实验误差的可接受范围内。图12中黑线为实验结果,蓝线为数值模拟结果,可以看出,初始冲击速度平台时长持续2 ns左右,准等熵加载段时长持续12 ns左右,实验曲线与数值模拟曲线在上升趋势上吻合良好,达到了相同的峰值,并且,相较于Al靶厚度为5 μm的情况,Al靶厚度为60 μm时数值模拟的粒子速度曲线的加载波形更为平滑,展现出良好的准等熵加载效果。
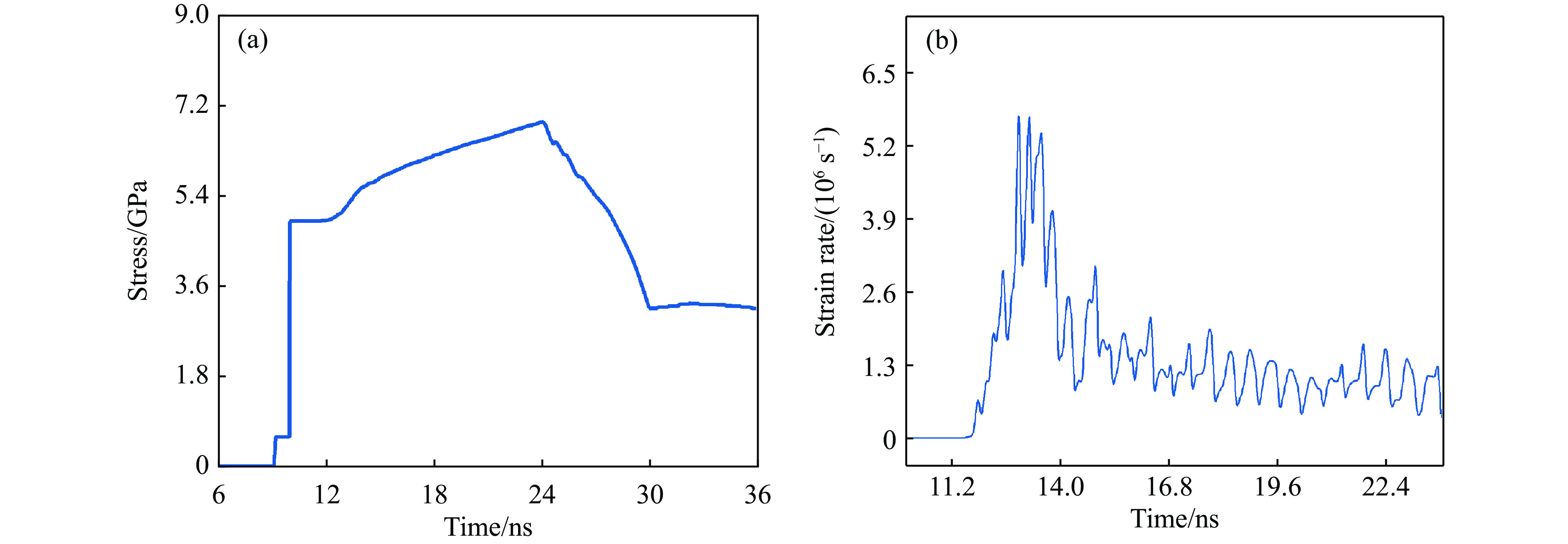
图13为数值模拟的Ti-Pt周期调制梯度材料在撞击60 μm厚Al靶过程中的应力和应变率与时间的关系。从图13(a)的应力曲线可以看出,Ti-Pt周期调制梯度材料以541.6 m/s速度撞击60 μm 厚Al靶时,应力从初始4.8 GPa连续上升至峰值应力6.9 GPa,波形为平滑的加载曲线。图13 (b)中,60 μm厚Al靶情况下的应变率均为正值,最大应变率为5.7×106 s−1,最小应变率为4.5×105 s−1,平均应变率为1.8×106 s−1。这一结果表明,Ti-Pt周期调制梯度材料撞击60 μm厚Al靶过程中应变率的振荡幅度显著减小,具有良好的准等熵加载效果。
3. 结 论
针对粉末冶金法制备的叠层组分梯度材料容易形成裂纹、孔洞、杂相、低平行度、低平整度以及金属合金相等问题,采用电子束蒸发镀膜技术成功制备出Ti-Pt周期调制梯度材料。该材料由30个1 μm的周期层组成,通过周期层内双组分(Ti与Pt单层)的厚度调控实现了宏观波阻抗的梯度变化。梯度材料的实测总厚度误差和不同周期层的平均波阻抗误差分别为1.67%和2.4%,层间界面清晰,物相分析未发现金属合金相,材料的平均硬度及弹性模量分别为2.8和99.8 GPa,即材料具有优异的力学性能。周期调制梯度结构能有效解决叠层组分梯度材料制备过程中的诸多问题。
当Ti-Pt周期调制梯度材料以480.9 m/s的速度撞击5 μm厚Al靶时,实验测得的粒子速度曲线与数值模拟结果在上升趋势上吻合良好。数值模拟结果表明,靶材在5 μm处的应力从初始的4 GPa持续上升至峰值应力5.5 GPa,应变率曲线在正负值间持续振荡,且幅度较大,最大应变率为7.3×107 s−1,最小应变率为−4.5×107 s−1,平均应变率为1.3×106 s−1。Ti-Pt周期调制梯度材料在加载过程中多个波系会相互追赶、叠加、整合,靶材在5 μm处的应力并不是单调上升,该位置处多道压缩波与稀疏波共同作用,应力先上升后下降再上升。靶材厚度对于完成波系整合和形成平滑的准等熵加载至关重要,将Al靶厚度从5 μm 增大至60 μm后,应力云图显示靶板在60 μm处波系完成整合。当Ti-Pt周期调制梯度材料以541.6 m/s的速度撞击60 μm厚Al靶时,不同于5 μm时的振荡上升波形,60 μm处Al靶的粒子速度与应力曲线转变为平滑的加载波形,应变率整体处于正值,最大应变率为5.7×106 s−1,最小应变率为4.5×105 s−1,平均应变率为1.8×106 s−1,具有良好的准等熵加载效果。研究结果可为新型周期调制梯度结构的应用提供指导。
-
图 4 Ti-Pt周期调制梯度材料的准等熵加载实验设计:(a) Ti-Pt周期调制梯度材料撞击Al靶示意图,(b) 粘结Ti-Pt周期调制梯度材料的弹托,(c) LiF窗口背面分布的光纤测速点
Figure 4. Experimental design of Ti-Pt periodically modulated gradient material: (a) schematic diagram of Ti-Pt periodically modulated gradient material impacting Al target; (b) a projectile bonded with Ti-Pt periodically modulated gradient material; (c) the distribution of velocity measurement fiber on the back of LiF window
图 6 Ti-Pt周期调制梯度材料的截面形貌(a),富Ti区的形貌(b),富Pt区的截面形貌(c), 能谱分析图(d),理论与实际波阻抗随位置的分布曲线(e)
Figure 6. Overall view (a); Ti-rich region view (b); Pt-rich region view (c); energy spectrum analysis diagram (d); theoretical and practical wave impedance distribution curve with position (e) for the Ti-Pt periodically modulated gradient material
图 11 Ti-Pt周期调制梯度材料撞击Al靶时的应力云图:(a) 0~120 μm区间内的应力云图,(b) 38~47 μm区间内的应力细节云图,(c) 90~110 μm区间内的应力云图
Figure 11. Stress contour map during Ti-Pt periodically modulated gradient material impacting Al target: (a) stress contour map from 0 to 120 μm; (b) detailed stress contour map from 38 μm to 47 μm; (c) detailed stress contour map from 90 μm to 110 μm
-
[1] HUANG H J, FEI Y W, CAI L C, et al. Evidence for an oxygen-depleted liquid outer core of the Earth [J]. Nature, 2011, 479(7374): 513–516. doi: 10.1038/nature10621 [2] NAKAJIMA Y, IMADA S, HIROSE K, et al. Carbon-depleted outer core revealed by sound velocity measurements of liquid iron-carbon alloy [J]. Nature Communications, 2015, 6(1): 8942. doi: 10.1038/ncomms9942 [3] SMITH R F, EGGERT J H, SWIFT D C, et al. Time-dependence of the alpha to epsilon phase transformation in iron [J]. Journal of Applied Physics, 2013, 114(22): 223507. doi: 10.1063/1.4839655 [4] VOGLER T J. On measuring the strength of metals at ultrahigh strain rates [J]. Journal of Applied Physics, 2009, 106(5): 053530. doi: 10.1063/1.3204777 [5] MILLOT M, COPPARI F, RYGG J R, et al. Nanosecond X-ray diffraction of shock-compressed superionic water ice [J]. Nature, 2019, 569(7755): 251–255. doi: 10.1038/s41586-019-1114-6 [6] SINGH A, SHIVLING V D, KHOSLA P K, et al. Strain hardening and shock mitigation response of polyurethane under high strain rates [J]. AIP Advances, 2021, 11(11): 115306. doi: 10.1063/5.0068403 [7] 谭华, 俞宇颖. 超高应变率准等熵加载和冲击加载下金属材料的强度 [J]. 高能量密度物理, 2010(3/4): 97–120. [8] NGUYEN J H, ORLIKOWSKI D, STREITZ F H, et al. High-pressure tailored compression: controlled thermodynamic paths [J]. Journal of Applied Physics, 2006, 100(2): 023508. doi: 10.1063/1.2214209 [9] BROWN N P, SPECHT P E, BROWN J L. Quasi-isentropic compression of an additively manufactured aluminum alloy to 14.8 GPa [J]. Journal of Applied Physics, 2022, 132(22): 225106. doi: 10.1063/5.0127989 [10] SHU H, LI J T, TU Y C, et al. Measurement of the sound velocity of shock compressed water [J]. Scientific Reports, 2021, 11(1): 6116. doi: 10.1038/s41598-021-84978-0 [11] MARTIN L P, PATTERSON J R, ORLIKOWSKI D, et al. Application of tape-cast graded impedance impactors for light-gas gun experiments [J]. Journal of Applied Physics, 2007, 102(2): 023507. doi: 10.1063/1.2756058 [12] TAN Y, LI X M, YU Y Y, et al. Research on phase transition and strength under ramp compression with graded density impactor [J]. EPJ Web of Conferences, 2018, 183: 02058. doi: 10.1051/epjconf/201818302058 [13] BASTEA M, REISMAN D B. Ramp compression experiments―a sensitivity study [J]. AIP Conference Proceedings, 2007, 955(1): 1169–1172. doi: 10.1063/1.2832927 [14] KELLY J P, NGUYEN J H, LIND J, et al. Application of Al-Cu-W-Ta graded density impactors in dynamic ramp compression experiments [J]. Journal of Applied Physics, 2019, 125(14): 145902. doi: 10.1063/1.5055398 [15] BROWN J L, ADAMS D P, ALEXANDER C S, et al. Estimates of Ta strength at ultrahigh pressures and strain rates using thin-film graded-density impactors [J]. Physical Review B, 2019, 99(21): 214105. doi: 10.1103/PhysRevB.99.214105 [16] RAY A, SINGLA A. Development of semiempirical equation of state of binary functionally graded materials and its influence on generation of ramp compression: comparison with bilayer graded density impactors [J]. Physical Review B, 2021, 103(5): 054301. doi: 10.1103/PhysRevB.103.054301 [17] 沈强, 张联盟, 王传彬, 等. 梯度飞片材料的波阻抗分布设计与优化 [J]. 物理学报, 2003, 52(7): 1663–1667. doi: 10.7498/aps.52.1663SHEN Q, ZHANG L M, WANG C B, et al. Design and optimization of wave impedance distribution for flyer materials [J]. Acta Physica Sinica, 2003, 52(7): 1663–1667. doi: 10.7498/aps.52.1663 [18] 刘石豪, 张振海, 牛兰杰, 等. 高能量冲击加载试验装置研究进展 [J]. 探测与控制学报, 2023, 45(4): 14–28.LIU S H, ZHANG Z H, NIU L J, et al. A Review on high-energy impact loading test device research [J]. Journal of Detection & Control, 2023, 45(4): 14–28. [19] ROGERS J A, BASS N T, WIEST M L, et al. The pursuit of hypervelocities: a review of two-stage light gas gun aeroballistic ranges [J]. International Journal of Impact Engineering, 2024, 185: 104861. doi: 10.1016/j.ijimpeng.2023.104861 [20] ZHOU Y H, TAN Y, ZHANG R Z, et al. Dynamics of stress waves in graded density impactors during the internal ballistic process [J]. Journal of Applied Physics, 2023, 134(22): 225901. doi: 10.1063/5.0177300 [21] DEWAELE A, STUTZMANN V, BOUCHET J, et al. High pressure-temperature phase diagram and equation of state of titanium [J]. Physical Review B, 2015, 91(13): 134108. doi: 10.1103/PhysRevB.91.134108 [22] ZHA C S, MIBE K, BASSETT W A, et al. P-V-T equation of state of platinum to 80 GPa and 1 900 K from internal resistive heating/X-ray diffraction measurements [J]. Journal of Applied Physics, 2008, 103(5): 054908. doi: 10.1063/1.2844358 [23] MARSH S P. LASL shock Hugoniot data [M]. Berkeley: University of California Press, 1980. [24] 徐锡申, 张万箱. 实用物态方程理论导引 [M]. 北京: 科学出版社, 1986. [25] MEYERS M A. Dynamic behavior of materials [M]. New York: John Wiley & Sons Inc., 1994. -








 下载:
下载:









 下载:
下载: